Use The Graphs To Evaluate The Expressions Below
Holbox
Mar 26, 2025 · 6 min read
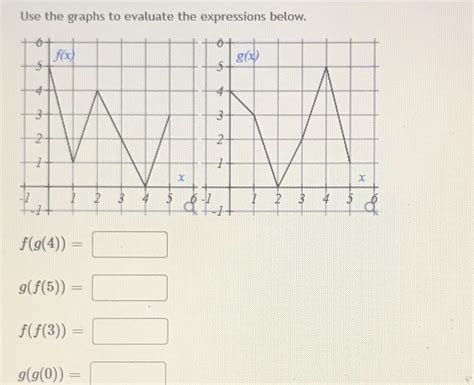
Table of Contents
- Use The Graphs To Evaluate The Expressions Below
- Table of Contents
- Using Graphs to Evaluate Expressions: A Comprehensive Guide
- Understanding the Relationship Between Graphs and Expressions
- Evaluating Linear Expressions Using Graphs
- Evaluating Quadratic Expressions Using Graphs
- Evaluating Other Types of Expressions Graphically
- Practical Applications
- Limitations of Graphical Evaluation
- Conclusion
- Latest Posts
- Latest Posts
- Related Post
Using Graphs to Evaluate Expressions: A Comprehensive Guide
Graphs are powerful visual tools that offer more than just a pretty picture of data. They provide a dynamic way to understand and evaluate mathematical expressions, offering insights that purely algebraic methods might miss. This comprehensive guide delves into the art of using graphs to evaluate expressions, covering various types of graphs and expressions, and highlighting practical applications.
Understanding the Relationship Between Graphs and Expressions
Before diving into specific examples, it's crucial to understand the fundamental connection between graphs and expressions. An expression, in its simplest form, is a mathematical phrase that combines numbers, variables, and operators. A graph, on the other hand, is a visual representation of the relationship between these variables. By plotting points derived from the expression, we create a visual representation of its behavior. The graph effectively shows us what the expression does.
This connection is particularly useful when dealing with expressions involving more than one variable. For example, while algebraic manipulation can tell us the solution to an equation like y = 2x + 1, a graph visually demonstrates the relationship between x and y for all possible values of x. This visual representation offers a broader, more intuitive understanding.
Evaluating Linear Expressions Using Graphs
Linear expressions, characterized by their constant rate of change, are easily evaluated graphically. These expressions are typically in the form y = mx + c, where m represents the slope (rate of change) and c represents the y-intercept (the point where the line crosses the y-axis).
Example: Let's consider the linear expression y = 3x + 2.
Steps to Evaluate Graphically:
-
Identify Key Points: The y-intercept is (0, 2). To find another point, choose a value for
x(e.g., x = 1). Substituting into the equation, we gety = 3(1) + 2 = 5. This gives us the point (1, 5). -
Plot the Points: Plot the points (0, 2) and (1, 5) on a Cartesian coordinate system.
-
Draw the Line: Draw a straight line through these points. This line represents the graph of the expression
y = 3x + 2. -
Evaluate the Expression: To evaluate the expression for a specific value of
x, find the correspondingyvalue on the graph. For example, ifx = 2, find the point on the line wherex = 2. The y-coordinate of that point will be the value of the expression whenx = 2. In this case, it would be 8 (because 3*2 + 2 = 8).
Advantages of Graphical Evaluation:
- Intuitive Understanding: The graph clearly shows the relationship between
xandy. - Multiple Evaluations: The graph allows quick evaluation for multiple values of
xwithout repetitive calculations. - Visualizing Rate of Change: The slope of the line visually represents the rate of change in
ywith respect tox.
Evaluating Quadratic Expressions Using Graphs
Quadratic expressions, represented by equations of the form y = ax² + bx + c (where a, b, and c are constants and a ≠ 0), create parabolic curves when graphed. Evaluating these expressions graphically involves similar steps, but with an added layer of complexity due to the curved nature of the graph.
Example: Let's consider the quadratic expression y = x² - 4x + 3.
Steps to Evaluate Graphically:
-
Find the Vertex: The vertex of a parabola is the point where the curve changes direction. For quadratic expressions in the standard form, the x-coordinate of the vertex is given by
x = -b/2a. In this case,x = -(-4)/2(1) = 2. Substituting this value into the equation, we find the y-coordinate:y = 2² - 4(2) + 3 = -1. The vertex is (2, -1). -
Find the x-intercepts: The x-intercepts are the points where the parabola crosses the x-axis (where y = 0). We can find these by solving the quadratic equation
x² - 4x + 3 = 0. Factoring, we get (x - 1)(x - 3) = 0, giving x-intercepts at (1, 0) and (3, 0). -
Find the y-intercept: The y-intercept is the point where the parabola crosses the y-axis (where x = 0). In this case,
y = 0² - 4(0) + 3 = 3, giving the y-intercept at (0, 3). -
Plot the Points and Draw the Parabola: Plot the vertex, x-intercepts, and y-intercept, then draw a smooth parabola through these points.
-
Evaluate the Expression: To evaluate the expression for a specific value of
x, find the correspondingyvalue on the parabola.
Advantages of Graphical Evaluation for Quadratic Expressions:
- Visualizing the Parabola's Shape: The graph immediately shows the parabola's concavity (whether it opens upwards or downwards) and its overall shape.
- Finding the Maximum or Minimum Value: The y-coordinate of the vertex represents the maximum or minimum value of the quadratic expression.
- Determining the Roots: The x-intercepts visually represent the roots (solutions) of the quadratic equation.
Evaluating Other Types of Expressions Graphically
The principles discussed above can be extended to evaluate other types of expressions, including:
- Cubic and Higher-Order Polynomials: These expressions produce curves with more complex shapes, but the basic principle of plotting points and interpreting the graph remains the same.
- Exponential and Logarithmic Functions: These functions exhibit exponential growth or decay and are characterized by their asymptotic behavior. Graphical evaluation helps visualize these characteristics.
- Trigonometric Functions: These functions describe periodic relationships and their graphs reveal the amplitude, period, and phase shift of the function.
Practical Applications
The ability to evaluate expressions graphically is valuable in various fields:
- Physics: Graphing equations of motion helps visualize the trajectory of projectiles or the relationship between velocity and time.
- Engineering: Graphical analysis of circuit equations helps understand current flow and voltage distribution.
- Economics: Graphing supply and demand curves helps analyze market equilibrium.
- Data Science: Visualizing data using graphs is fundamental to understanding trends and patterns in datasets.
Limitations of Graphical Evaluation
While graphical evaluation offers valuable insights, it has limitations:
- Accuracy: The accuracy of graphical evaluation depends on the precision of the graph and the ability to accurately read values from it. For precise calculations, algebraic methods are often preferred.
- Complexity: Complex expressions may be difficult to graph accurately by hand, requiring specialized software.
- Non-continuous Functions: Functions with discontinuities or asymptotes require careful interpretation of the graph.
Conclusion
Using graphs to evaluate expressions offers a powerful alternative to purely algebraic methods. It provides a visual and intuitive understanding of the relationship between variables, especially when dealing with multiple variables or complex functions. While not always the most precise method, graphical evaluation's strength lies in its ability to provide a broader, more insightful understanding of the expression's behavior. Combining graphical analysis with algebraic methods provides a robust and comprehensive approach to evaluating mathematical expressions. Mastering both techniques equips you with powerful tools for problem-solving in various scientific and mathematical contexts.
Latest Posts
Latest Posts
-
What Should Managers Do When It Comes To Stress Reduction
Mar 29, 2025
-
Draw The Major Organic Product Of The Following Reaction Sequence
Mar 29, 2025
-
For Each Market Determine Whether Each Market
Mar 29, 2025
-
The Sister Chromatids Are Moving Apart
Mar 29, 2025
-
Consider The Following Matrices Find The Matrix Such That
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about Use The Graphs To Evaluate The Expressions Below . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
