A Block Initially At Rest Is Given A Quick Push
Holbox
Mar 27, 2025 · 7 min read
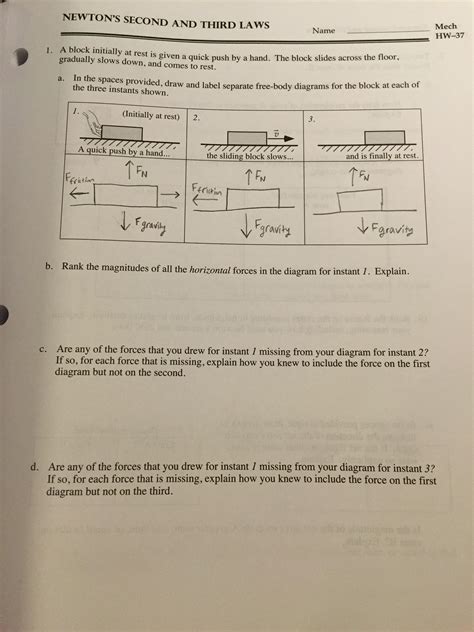
Table of Contents
- A Block Initially At Rest Is Given A Quick Push
- Table of Contents
- A Block Initially at Rest: Exploring the Physics of Impulse and Motion
- Understanding Impulse: The Driving Force Behind Motion
- The Role of Force and Time in Impulse
- Momentum: The Measure of Motion
- Friction: The Unseen Opponent
- Static Friction: Resistance to Initial Movement
- Kinetic Friction: Resistance During Movement
- The Influence of Surface Properties
- Mathematical Modeling of the Block's Motion
- Kinematic Equations
- Advanced Considerations: Beyond the Basics
- Conclusion: A Simple Act, Complex Physics
- Latest Posts
- Latest Posts
- Related Post
A Block Initially at Rest: Exploring the Physics of Impulse and Motion
Have you ever pushed a stationary object, like a block? It's a seemingly simple act, yet it reveals fundamental principles of physics. This article delves deep into the physics behind a block initially at rest that receives a quick push, exploring concepts like impulse, momentum, friction, and the impact of different surfaces. We'll use this seemingly simple scenario to understand complex physics concepts and delve into the mathematical descriptions that govern this movement.
Understanding Impulse: The Driving Force Behind Motion
When you give a block a quick push, you're not just applying force; you're applying a force over a specific time interval. This combination of force and time is known as impulse. Impulse is a vector quantity, meaning it has both magnitude and direction. Mathematically, impulse (J) is represented as:
J = FΔt
where:
- J represents the impulse
- F represents the average force applied
- Δt represents the change in time (the duration of the push)
The crucial takeaway here is that a larger impulse leads to a greater change in the block's momentum. A stronger push (larger F) or a longer push (larger Δt) both contribute to a larger impulse and thus a larger change in motion. This is pivotal in understanding why a quick, strong push imparts a significant velocity compared to a gentle, prolonged push.
The Role of Force and Time in Impulse
Let's consider two scenarios:
-
Scenario 1: Strong push, short time: Imagine a powerful, quick shove. The force (F) is high, but the time (Δt) is short. However, the product FΔt (the impulse) can still be substantial, resulting in a significant change in the block's momentum and velocity.
-
Scenario 2: Gentle push, long time: Now, picture pushing the block gently but continuously for a longer duration. The force (F) is low, but the time (Δt) is long. If the product FΔt (the impulse) is comparable to the first scenario, the change in the block's momentum will be similar, even if the experience feels different.
This highlights the interconnectedness of force and time in determining the overall impact of the push.
Momentum: The Measure of Motion
Momentum (p) is another crucial concept. It’s a measure of an object's mass in motion. It's a vector quantity, meaning it has both magnitude and direction. The formula for momentum is:
p = mv
where:
- p represents momentum
- m represents the mass of the block
- v represents the velocity of the block
The impulse-momentum theorem elegantly connects impulse and momentum:
J = Δp = mv<sub>f</sub> - mv<sub>i</sub>
where:
- mv<sub>f</sub> is the final momentum
- mv<sub>i</sub> is the initial momentum (which is 0 for a block at rest)
This theorem states that the impulse acting on an object is equal to the change in its momentum. This means the push changes the block's momentum from zero to a non-zero value. A larger impulse results in a larger change in momentum, translating to a higher final velocity.
Friction: The Unseen Opponent
Once the push ends, the block doesn't continue moving indefinitely. Friction plays a significant role. Friction opposes the motion of the block against the surface it rests upon. There are two main types of friction to consider:
Static Friction: Resistance to Initial Movement
Static friction prevents the block from moving initially. It's the force that needs to be overcome before the block starts to slide. The maximum static friction force (f<sub>s</sub>) is given by:
f<sub>s</sub> ≤ μ<sub>s</sub>N
where:
- μ<sub>s</sub> is the coefficient of static friction (a property of the surfaces in contact)
- N is the normal force (the force exerted by the surface perpendicular to the block, typically equal to the weight of the block, mg, on a horizontal surface)
The block will only start moving if the applied force during the push exceeds the maximum static friction force.
Kinetic Friction: Resistance During Movement
Once the block starts moving, kinetic friction (also called sliding friction) continues to oppose its motion. The kinetic friction force (f<sub>k</sub>) is given by:
f<sub>k</sub> = μ<sub>k</sub>N
where:
- μ<sub>k</sub> is the coefficient of kinetic friction (typically smaller than μ<sub>s</sub>)
Kinetic friction slows the block down until it eventually comes to rest. The difference between static and kinetic friction explains why it often takes more force to start an object moving than to keep it moving.
The Influence of Surface Properties
The nature of the surfaces involved significantly impacts the motion of the block. A smooth, polished surface will exhibit lower coefficients of friction (both static and kinetic) compared to a rough, textured surface. This translates to:
- Less resistance to initial movement: The block will start moving more easily on a smooth surface.
- Less deceleration after the push: The block will travel farther on a smooth surface before coming to rest.
Conversely, a rough surface will result in greater friction, leading to more resistance to initial movement and faster deceleration after the push.
Mathematical Modeling of the Block's Motion
To accurately describe the block's motion, we can utilize Newton's second law of motion:
F<sub>net</sub> = ma
where:
- F<sub>net</sub> is the net force acting on the block.
- m is the mass of the block.
- a is the acceleration of the block.
During the push, F<sub>net</sub> is the difference between the applied force and the static friction force (until the block starts moving), then the difference between the applied force and the kinetic friction force (once the block is moving). After the push, F<sub>net</sub> is simply the negative of the kinetic friction force. Solving this equation, combined with kinematic equations, allows us to determine the block's velocity and displacement at any point in time.
Kinematic Equations
The following kinematic equations are crucial for determining the block's motion:
- v<sub>f</sub> = v<sub>i</sub> + at (relates final velocity, initial velocity, acceleration, and time)
- Δx = v<sub>i</sub>t + ½at² (relates displacement, initial velocity, acceleration, and time)
- v<sub>f</sub>² = v<sub>i</sub>² + 2aΔx (relates final velocity, initial velocity, acceleration, and displacement)
By using these equations along with Newton's second law, we can model the block's motion with reasonable accuracy, assuming constant forces and neglecting other factors like air resistance.
Advanced Considerations: Beyond the Basics
The analysis above provides a fundamental understanding. However, several factors could influence the block's motion in a real-world scenario:
-
Air Resistance: Air resistance opposes the block's motion, especially at higher velocities. It depends on factors like the block's shape, size, and the density of the air.
-
Non-uniform Surfaces: If the surface is uneven, the frictional forces will vary, making the analysis significantly more complex.
-
Elasticity: If the block and the surface are slightly elastic, some energy will be lost to deformation during the collision, slightly reducing the final velocity.
-
Rolling Friction: If the block is not perfectly rigid and has rounded edges or a cylindrical shape, then it might undergo rolling motion rather than simple sliding, requiring additional considerations of rolling friction.
-
Angle of Inclination: If the surface is not perfectly horizontal, the gravitational force component parallel to the surface will interact with the applied force and frictional forces, influencing the resultant motion.
Conclusion: A Simple Act, Complex Physics
The seemingly simple act of pushing a block initially at rest reveals a rich tapestry of physical concepts. By understanding impulse, momentum, friction, and the properties of surfaces, we can predict and analyze the block's motion with increasing accuracy. The principles illustrated here are fundamental to understanding many other aspects of classical mechanics, from projectile motion to the impact of collisions. This foundational knowledge paves the way to grasping more intricate scenarios and the complexities of real-world systems.
Latest Posts
Latest Posts
-
Which Of The Following Is The Strongest Acid
Mar 31, 2025
-
Please Label The Circular Flow Diagram
Mar 31, 2025
-
Identify True Statements About The Si Unit Of Force
Mar 31, 2025
-
All Of The Following Are True About Sql Except
Mar 31, 2025
-
One Page Memo Between Fraud And Internal Controls
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about A Block Initially At Rest Is Given A Quick Push . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
