Identify True Statements About The Si Unit Of Force.
Holbox
Mar 31, 2025 · 6 min read
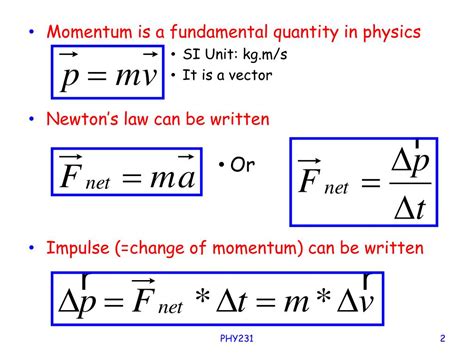
Table of Contents
- Identify True Statements About The Si Unit Of Force.
- Table of Contents
- Identify True Statements About the SI Unit of Force
- Defining the Newton: A Deep Dive
- True Statements Regarding the Definition:
- Exploring the Applications of the Newton
- True Statements Regarding Applications:
- Relationship with Other Units: Pascal and Joule
- True Statements Regarding Related Units:
- Common Misconceptions About the Newton
- Conclusion: Mastering the Newton
- Latest Posts
- Latest Posts
- Related Post
Identify True Statements About the SI Unit of Force
The SI unit of force, the newton (N), is a fundamental concept in physics and engineering. Understanding its definition and applications is crucial for accurate calculations and problem-solving. This comprehensive guide will delve into the intricacies of the newton, identifying true statements about it and dispelling common misconceptions. We'll explore its relationship with mass, acceleration, and other relevant physical quantities, providing a solid foundation for anyone working with forces and mechanics.
Defining the Newton: A Deep Dive
The newton is defined as the amount of force required to accelerate a mass of one kilogram at a rate of one meter per second squared. This seemingly simple definition encapsulates a profound relationship between force, mass, and acceleration, as elegantly expressed by Newton's second law of motion: F = ma, where:
- F represents force (measured in newtons)
- m represents mass (measured in kilograms)
- a represents acceleration (measured in meters per second squared)
This equation forms the bedrock of classical mechanics and is essential for understanding how forces affect the motion of objects. It's crucial to remember that this is a vector equation; both force and acceleration have both magnitude and direction. A force acting in one direction will cause an acceleration in that same direction.
True Statements Regarding the Definition:
-
Statement 1: A newton is the force required to accelerate a 1 kg mass by 1 m/s². This is the direct and fundamental definition of the newton. It's the cornerstone upon which all other understandings of the unit are built.
-
Statement 2: The newton is a derived unit, not a base unit. Unlike the kilogram (mass), second (time), and meter (length), which are base SI units, the newton is derived from these base units. Its derivation is directly evident from F = ma.
-
Statement 3: The dimensions of a newton are kg⋅m/s². This follows directly from the equation F = ma. The units of mass (kg), acceleration (m/s²), are combined to give the unit of force, the newton (kg⋅m/s²). Understanding the dimensional analysis of the newton is vital for ensuring consistency in calculations and for performing dimensional checks to verify the accuracy of equations.
Exploring the Applications of the Newton
The newton finds extensive application across various fields, including:
-
Classical Mechanics: Newton's second law (F = ma) is the workhorse of classical mechanics. It allows us to analyze the motion of objects subjected to various forces, such as gravity, friction, and applied forces.
-
Engineering: Engineers use the newton to design structures, machines, and systems that can withstand forces and stresses. This is crucial in ensuring structural integrity and safety in various applications, from bridges and buildings to aircraft and spacecraft.
-
Fluid Mechanics: The newton is used to describe forces within fluids, including pressure, buoyancy, and drag. Understanding these forces is essential for designing efficient systems involving fluid flow, like pipelines, pumps, and aerodynamic vehicles.
-
Material Science: The newton is used to determine material strength and elasticity. Tensile strength, compressive strength, and shear strength are all expressed in terms of force per unit area (pascals), which are derived from the newton.
True Statements Regarding Applications:
-
Statement 4: The weight of an object is a force measured in newtons. Weight is the force exerted on an object due to gravity. On Earth, an object with a mass of 1 kg experiences a gravitational force of approximately 9.8 N (weight = mass x gravitational acceleration).
-
Statement 5: The newton is used to calculate work and energy. Work is the product of force and displacement (W = Fd). Energy, being the capacity to do work, is also measured in joules (J), which are equivalent to newton-meters (N⋅m).
-
Statement 6: The newton is used in calculating momentum. Momentum (p) is the product of mass and velocity (p = mv). The change in momentum is directly proportional to the force applied and the time over which it acts (impulse-momentum theorem).
-
Statement 7: The newton is applicable to both macroscopic and microscopic systems. While often used in macroscopic systems (e.g., analyzing the motion of cars or planets), the newton also applies to microscopic systems, albeit often requiring more sophisticated calculations and considering other forces like electromagnetic forces.
Relationship with Other Units: Pascal and Joule
The newton is intrinsically linked to other important SI units:
-
Pascal (Pa): The pascal is the SI unit of pressure, defined as one newton per square meter (N/m²). Pressure is force distributed over an area, making the pascal a derived unit directly related to the newton. High pressures, such as those found in hydraulic systems or deep within the Earth, involve significant forces expressed in newtons distributed over specific areas.
-
Joule (J): The joule is the SI unit of energy and work. It is defined as one newton-meter (N⋅m). Work done is the product of the force applied and the distance the object moves in the direction of the force. Energy, in its many forms (kinetic, potential, etc.), is always expressed in joules and its connection to the newton demonstrates the inherent link between force and energy.
True Statements Regarding Related Units:
-
Statement 8: A newton-meter is equivalent to a joule. This highlights the fundamental connection between force, displacement, and energy. Lifting a 10-newton weight one meter requires 10 joules of work.
-
Statement 9: Pressure is force per unit area, measured in pascals (N/m²). This establishes the connection between force and pressure. A larger force concentrated on a smaller area results in higher pressure.
-
Statement 10: The newton is a vector quantity. This emphasizes that the newton has both magnitude (the numerical value) and direction (the orientation of the force). This aspect is crucial for correctly applying Newton's laws and solving complex problems involving multiple forces acting on an object.
Common Misconceptions About the Newton
It is important to address some common misconceptions surrounding the newton:
-
Misconception 1: The newton is only used for gravitational forces. While gravity is a significant force and is often used in examples, the newton applies to all types of forces, including friction, tension, electromagnetic forces, and more.
-
Misconception 2: Mass and weight are the same thing. Mass is a measure of an object's inertia (resistance to change in motion), while weight is the force of gravity acting on that mass. They are related (weight = mass x gravitational acceleration), but are distinct concepts with different units (kilograms for mass, newtons for weight).
-
Misconception 3: The newton is only relevant in physics. The newton is a fundamental unit used across various scientific and engineering disciplines, from mechanical engineering to aerospace engineering to material science.
Conclusion: Mastering the Newton
The newton, the SI unit of force, is a cornerstone of classical mechanics and a fundamental concept across various scientific and engineering fields. Understanding its definition, applications, and relationships with other units is crucial for solving problems and building a strong foundation in physics and engineering. By correctly identifying true statements about the newton and dispelling common misconceptions, we can appreciate the power and versatility of this fundamental unit of measurement. This comprehensive exploration aimed to clarify the key aspects of the newton, leaving readers with a confident and well-rounded understanding of this critical concept. Remember, grasping the fundamental concepts, like the definition and applications of the newton, is key to unlocking deeper understanding and problem-solving abilities in the realm of physics and beyond.
Latest Posts
Latest Posts
-
Which Code Example Is An Expression
Apr 03, 2025
-
Good Team Goals Are All Of The Following Except
Apr 03, 2025
-
During The Year Trc Corporation Has The Following Inventory Transactions
Apr 03, 2025
-
In Project Network Analysis Slack Refers To The Difference Between
Apr 03, 2025
-
The Economic Way Of Thinking Stresses That
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Identify True Statements About The Si Unit Of Force. . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
