___ Refers To Output Given A Set Of Resources/inputs.
Holbox
Mar 24, 2025 · 6 min read
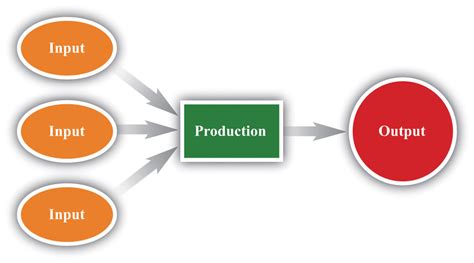
Table of Contents
- ___ Refers To Output Given A Set Of Resources/inputs.
- Table of Contents
- Production Function: Understanding Output from Inputs
- What is a Production Function?
- Types of Production Functions
- 1. Linear Production Function
- 2. Cobb-Douglas Production Function
- 3. Leontief Production Function (Fixed Proportions)
- 4. CES (Constant Elasticity of Substitution) Production Function
- Factors Affecting Production Functions
- 1. Technology
- 2. Management Efficiency
- 3. Labor Skills and Training
- 4. Quality of Inputs
- Applications of Production Functions
- 1. Business Decision-Making
- 2. Economic Policy
- 3. Agricultural Economics
- 4. Manufacturing and Operations Management
- Limitations of Production Functions
- Conclusion: The Importance of Understanding Production Functions
- Latest Posts
- Latest Posts
- Related Post
Production Function: Understanding Output from Inputs
The term production function refers to the output given a set of resources/inputs. It's a fundamental concept in economics that describes the relationship between the quantity of inputs used in production and the quantity of output produced. Understanding the production function is crucial for businesses to optimize their operations, maximize profits, and make informed decisions about resource allocation. This comprehensive guide delves deep into the intricacies of production functions, exploring various types, influencing factors, and their practical applications.
What is a Production Function?
At its core, a production function is a mathematical representation of the technological relationship between inputs and outputs. It shows the maximum amount of output that can be produced from a given set of inputs, assuming efficient use of those resources. This function doesn't account for market prices or the economic feasibility of using those inputs; it simply focuses on the technical possibilities. A simple representation might look like this:
Q = f(L, K, M)
Where:
- Q represents the quantity of output.
- f represents the production function itself (the specific relationship between inputs and outputs).
- L represents labor (number of workers, hours worked).
- K represents capital (machinery, equipment, buildings).
- M represents materials (raw materials, intermediate goods).
This basic model can be expanded to include other inputs like land, energy, technology, and management expertise. The complexity of the function depends on the nature of the production process.
Types of Production Functions
Several types of production functions exist, each offering a different perspective on the relationship between inputs and outputs. The choice of function depends on the specific industry, technology, and the level of detail required for analysis.
1. Linear Production Function
This is the simplest form, where the output is directly proportional to the inputs. It assumes constant returns to scale, meaning that doubling the inputs doubles the output. Mathematically, it's represented as:
Q = aL + bK
where 'a' and 'b' are constants representing the marginal productivity of labor and capital, respectively. While simple, this function is rarely accurate in real-world scenarios as it neglects the complexities of diminishing returns.
2. Cobb-Douglas Production Function
This is a widely used and versatile function that incorporates diminishing returns to scale. It's expressed as:
Q = AL<sup>α</sup>K<sup>β</sup>
Where:
- A represents total factor productivity (reflecting technology and efficiency).
- α and β are the output elasticities of labor and capital, respectively. They represent the percentage change in output resulting from a 1% change in labor or capital, holding the other input constant. The sum of α and β determines the returns to scale:
- α + β = 1: Constant returns to scale.
- α + β < 1: Decreasing returns to scale.
- α + β > 1: Increasing returns to scale.
3. Leontief Production Function (Fixed Proportions)
This function assumes that inputs must be used in fixed proportions. It represents situations where inputs are complements and cannot be substituted for each other. A classic example is a recipe where the ingredients must be used in specific ratios. It's represented as:
Q = min(aL, bK)
The output is limited by the input that is in shortest supply.
4. CES (Constant Elasticity of Substitution) Production Function
This function allows for substitution between inputs, but the elasticity of substitution remains constant. It provides a more flexible framework than the Cobb-Douglas function, allowing for different degrees of substitutability between inputs. The general form is complex, but it allows for a wide range of production scenarios.
Factors Affecting Production Functions
Several factors influence the shape and parameters of the production function. These factors can shift the entire function or alter its slope, impacting the output for a given set of inputs.
1. Technology
Technological advancements significantly impact productivity. Improvements in technology can shift the production function upwards, allowing for more output with the same level of inputs or the same output with fewer inputs. This is captured in the 'A' term in the Cobb-Douglas function.
2. Management Efficiency
Effective management practices can improve resource allocation and coordination, leading to increased output. Better management can reduce waste, improve worker productivity, and optimize the use of capital equipment.
3. Labor Skills and Training
A skilled and well-trained workforce is more productive than an unskilled one. Investment in human capital through training and education can significantly improve the output for a given level of labor input.
4. Quality of Inputs
The quality of inputs directly affects the output. Using higher-quality raw materials or more reliable machinery can lead to a significant increase in output.
Applications of Production Functions
Understanding production functions has numerous practical applications in various fields:
1. Business Decision-Making
Businesses use production functions to determine the optimal combination of inputs to maximize output and profits. They can analyze the marginal productivity of each input and adjust their resource allocation accordingly.
2. Economic Policy
Governments use production functions to model the economy's overall productivity and to evaluate the impact of economic policies on output. They can analyze the effects of investments in infrastructure, education, and technology on economic growth.
3. Agricultural Economics
In agriculture, production functions are crucial for determining the optimal mix of inputs like fertilizers, seeds, water, and labor to maximize crop yields.
4. Manufacturing and Operations Management
Manufacturing firms employ production functions to optimize their production processes, minimize costs, and improve efficiency. They use them to plan production schedules, manage inventories, and allocate resources.
Limitations of Production Functions
While production functions are powerful tools, they have certain limitations:
-
Simplification: Real-world production processes are highly complex and difficult to fully capture in a simple mathematical function. Many factors are often omitted or simplified for analytical purposes.
-
Data Requirements: Accurate estimation of production functions requires reliable data on inputs and outputs, which can be challenging to obtain in practice.
-
Technological Change: Technological advancements can render existing production functions obsolete, requiring frequent updates and revisions.
-
External Factors: Production functions typically don't explicitly account for external factors such as market demand, government regulations, or unforeseen events (e.g., natural disasters).
Conclusion: The Importance of Understanding Production Functions
Despite their limitations, production functions remain a fundamental tool for understanding the relationship between inputs and outputs. They provide a framework for businesses and policymakers to analyze productivity, make informed decisions, and improve resource allocation. By understanding the different types of production functions and the factors that influence them, individuals and organizations can gain valuable insights into optimizing their operations and achieving their objectives. Furthermore, continuously monitoring and updating production function models in light of technological advancements and changing market conditions is vital for sustained success. The ongoing refinement and application of these models are key to economic growth and enhanced productivity across various sectors.
Latest Posts
Latest Posts
-
Experiment 17 Lewis Structures And Molecular Models Answers
Mar 29, 2025
-
You Have Recently Opened Your Own Internet Website Design
Mar 29, 2025
-
Xf Xi Vit 1 2at 2
Mar 29, 2025
-
The Use Of Buprenorphine Can Decrease And Increase
Mar 29, 2025
-
Art Labeling Activity Internal Midsagittal View Of The Skull
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about ___ Refers To Output Given A Set Of Resources/inputs. . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
