A Nonconducting Slab With Volume Charge Density
Holbox
Mar 26, 2025 · 5 min read
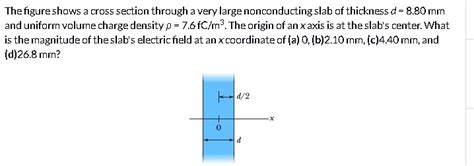
Table of Contents
- A Nonconducting Slab With Volume Charge Density
- Table of Contents
- A Nonconducting Slab with Volume Charge Density: A Comprehensive Exploration
- Understanding the Fundamentals: Charge Density and Gauss's Law
- Electric Field Inside and Outside a Uniformly Charged Slab
- Inside the Slab (-a < z < a):
- Outside the Slab (z > a or z < -a):
- Non-Uniform Charge Density: A More Complex Scenario
- Potential Difference and Potential Energy
- Potential Difference:
- Potential Energy:
- Applications and Further Exploration
- Latest Posts
- Latest Posts
- Related Post
A Nonconducting Slab with Volume Charge Density: A Comprehensive Exploration
The concept of a nonconducting slab with a volume charge density is a cornerstone in electrostatics, offering a rich platform to understand Gauss's law and the behavior of electric fields in continuous charge distributions. This article delves deep into this topic, exploring the electric field both inside and outside the slab, tackling various scenarios involving different charge density distributions, and finally extending the discussion to potential differences and potential energy considerations.
Understanding the Fundamentals: Charge Density and Gauss's Law
Before diving into the specifics of the nonconducting slab, let's establish a solid foundation. Volume charge density (ρ) represents the amount of charge per unit volume. It's typically expressed in Coulombs per cubic meter (C/m³). This is crucial because, unlike point charges, we're dealing with a continuous distribution of charge throughout the slab's volume.
Gauss's law provides the crucial link between charge distribution and the resulting electric field. It states that the flux of the electric field through any closed surface is proportional to the enclosed charge. Mathematically:
∮ E ⋅ dA = Q<sub>enc</sub> / ε₀
where:
- E is the electric field vector
- dA is a vector element of the surface area
- Q<sub>enc</sub> is the net charge enclosed within the surface
- ε₀ is the permittivity of free space (8.854 × 10⁻¹² C²/Nm²)
This seemingly simple equation is incredibly powerful because it allows us to calculate the electric field due to various charge distributions, including our nonconducting slab.
Electric Field Inside and Outside a Uniformly Charged Slab
Let's consider a nonconducting slab of thickness '2a' and infinite extent in the x and y directions. The slab possesses a uniform volume charge density ρ. This means the charge density is constant throughout the entire volume of the slab.
To determine the electric field, we employ Gauss's law. Due to symmetry, we choose a Gaussian surface – a rectangular box – that extends from the center of the slab symmetrically outwards.
Inside the Slab (-a < z < a):
Consider a point at a distance 'z' from the center of the slab. The enclosed charge within our Gaussian surface is given by:
Q<sub>enc</sub> = ρ * A * 2z
where 'A' is the area of the rectangular box's face perpendicular to the z-axis.
Applying Gauss's law:
EA = (ρ * A * 2z) / ε₀
Notice that the area 'A' cancels out, giving us the electric field inside the slab:
E<sub>inside</sub> = (ρz) / ε₀
This equation reveals that the electric field inside the slab is linearly proportional to the distance 'z' from the center. At the center (z=0), the electric field is zero, as expected due to symmetry.
Outside the Slab (z > a or z < -a):
For a point outside the slab, the enclosed charge is simply the total charge within the slab:
Q<sub>enc</sub> = ρ * A * 2a
Applying Gauss's law and following similar steps as above, we get:
E<sub>outside</sub> = (ρa) / ε₀
Importantly, the electric field outside the slab is constant and independent of the distance from the slab. Its direction is perpendicular to the slab's surface, pointing away from the slab if ρ is positive and towards the slab if ρ is negative.
Non-Uniform Charge Density: A More Complex Scenario
The assumption of uniform charge density simplifies calculations significantly. However, real-world scenarios might involve non-uniform charge distributions. Let's consider a scenario where the volume charge density is a function of the position within the slab, for example:
ρ(z) = ρ₀ (1 - z²/a²)
This represents a charge density that is maximum at the center (z=0) and decreases to zero at the edges (z = ±a).
Solving for the electric field in this case becomes significantly more challenging and often requires integration techniques. The basic principle remains the same: we still use Gauss's law, but the enclosed charge calculation becomes an integral:
Q<sub>enc</sub> = ∫ ρ(z) dA dz
The subsequent calculation of the electric field will involve solving this integral and applying Gauss's law to obtain the electric field expression as a function of 'z'. This calculation would likely involve solving a definite integral with limits determined by the position of the Gaussian surface relative to the slab. Numerical methods might be necessary for complex charge density functions.
Potential Difference and Potential Energy
Understanding the electric field allows us to calculate the potential difference (voltage) between two points and the potential energy of a charge within the electric field.
Potential Difference:
The potential difference (ΔV) between two points A and B is given by the line integral of the electric field:
ΔV = - ∫<sub>A</sub><sup>B</sup> E ⋅ dl
For the uniformly charged slab, this integral can be evaluated separately for the regions inside and outside the slab, leading to expressions for potential differences between different points within and outside the slab.
Potential Energy:
The potential energy (U) of a point charge 'q' placed in an electric potential V is simply:
U = qV
This allows us to determine the potential energy of a charge placed at any point within or outside the charged slab.
Applications and Further Exploration
The concept of a nonconducting slab with volume charge density finds numerous applications in various fields of physics and engineering. Some examples include:
- Capacitors: Dielectric materials within capacitors can be modeled as nonconducting slabs with polarization charges, influencing the capacitance and energy storage.
- Semiconductors: Charge distributions within semiconductor devices can be approximated using volume charge density models.
- Atmospheric Physics: The study of charge distribution in the atmosphere often involves modeling charge densities in various layers.
Further exploration of this topic could include:
- Investigating slabs with more complex geometries.
- Examining the effects of different boundary conditions.
- Exploring the behavior of conductors placed near or within the charged slab.
Understanding the electrostatics of a nonconducting slab is essential for grasping many fundamental concepts in physics and has profound implications for various applications. While the uniform charge density case provides a manageable introduction, tackling non-uniform distributions and more complex geometries demonstrates the depth and versatility of this concept. This article has aimed to provide a thorough and accessible overview, encouraging further investigation and a deeper understanding of this fascinating area of electrostatics.
Latest Posts
Latest Posts
-
The Evidence In Evidence Based Policing Refers To
Mar 29, 2025
-
A 78 Confidence Interval For A Proportion
Mar 29, 2025
-
Which Report Indicates When Quickbooks Users Signed In And Out
Mar 29, 2025
-
Based On The Above Income Statement Data And The Formula
Mar 29, 2025
-
The Largest Expense For Most Airlines Is
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about A Nonconducting Slab With Volume Charge Density . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
