1-2 Additional Practice Transformations Of Functions Answers
Holbox
Mar 31, 2025 · 5 min read
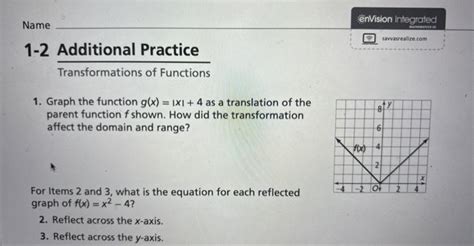
Table of Contents
- 1-2 Additional Practice Transformations Of Functions Answers
- Table of Contents
- 1-2 Additional Practice Transformations of Functions: Answers and Deep Dive
- Problem 1: Analyzing a Composite Transformation
- Solution: Deconstructing the Transformation
- Graphing g(x)
- Problem 2: Transforming a Square Root Function
- Solution: Step-by-Step Transformation Analysis
- Graphing k(x)
- General Strategies for Function Transformations
- Advanced Transformation Problems and Concepts
- Conclusion: Mastering Function Transformations
- Latest Posts
- Latest Posts
- Related Post
1-2 Additional Practice Transformations of Functions: Answers and Deep Dive
Transformations of functions are a cornerstone of algebra and precalculus, providing a powerful framework for understanding how changes to a function's equation affect its graph. This article delves into two additional practice problems focusing on function transformations, providing detailed solutions and explanations. We'll explore the core concepts involved – translations (shifts), reflections, and stretches/compressions – to solidify your understanding. Beyond the solutions, we'll examine the underlying principles and strategies to tackle any function transformation problem you encounter.
Problem 1: Analyzing a Composite Transformation
Let's consider the function f(x) = x². Describe the transformations applied to f(x) to obtain the function g(x) = -2(x + 3)² + 4. Then, sketch the graph of g(x), indicating key points like the vertex and intercepts.
Solution: Deconstructing the Transformation
The function g(x) is a transformed version of f(x) = x². To understand the transformations, we break down g(x) step-by-step:
-
Horizontal Shift: The term
(x + 3)indicates a horizontal shift of 3 units to the left. Remember that a "+3" inside the parentheses shifts to the left, while a "-3" would shift to the right. -
Vertical Stretch/Compression: The coefficient
-2acts as a vertical stretch by a factor of 2 and a reflection across the x-axis. The negative sign flips the parabola upside down. -
Vertical Shift: The "+4" at the end represents a vertical shift of 4 units upward.
Graphing g(x)
To sketch the graph, we start with the basic parabola f(x) = x². Applying the transformations sequentially:
- Shift left 3 units: The vertex moves from (0,0) to (-3,0).
- Stretch by 2 and reflect: The parabola becomes narrower and flips downwards.
- Shift up 4 units: The vertex moves from (-3,0) to (-3,4).
The vertex of g(x) is (-3, 4). To find the x-intercepts, we set g(x) = 0 and solve for x:
0 = -2(x + 3)² + 4 2(x + 3)² = 4 (x + 3)² = 2 x + 3 = ±√2 x = -3 ± √2
Therefore, the x-intercepts are approximately (-3 - √2, 0) and (-3 + √2, 0). The y-intercept is found by setting x = 0:
g(0) = -2(0 + 3)² + 4 = -18 + 4 = -14
The y-intercept is (0, -14). Using these key points, we can accurately sketch the parabola.
Problem 2: Transforming a Square Root Function
Consider the function h(x) = √x. Describe the transformations applied to h(x) to get k(x) = 3√(x - 2) + 1. Graph k(x), labeling key features.
Solution: Step-by-Step Transformation Analysis
Similar to the previous problem, we break down the transformations:
-
Horizontal Shift: The
(x - 2)term inside the square root indicates a horizontal shift of 2 units to the right. -
Vertical Stretch: The coefficient
3represents a vertical stretch by a factor of 3. -
Vertical Shift: The
+1at the end signifies a vertical shift of 1 unit upward.
Graphing k(x)
The graph of h(x) = √x starts at the origin (0,0) and increases gradually. Applying the transformations:
- Shift right 2 units: The starting point shifts from (0,0) to (2,0).
- Stretch vertically by 3: The graph becomes steeper.
- Shift up 1 unit: The starting point shifts from (2,0) to (2,1).
The starting point (or endpoint of the domain restriction) of k(x) is (2, 1). The graph extends infinitely to the right. To find the y-intercept, we set x = 0:
k(0) = 3√(0 - 2) + 1 (Note: this is not in the domain, so we know there is no y-intercept on the positive side)
Since the square root of a negative number is not a real number, there's no y-intercept in the real number system. This reinforces that the graph is only defined for x ≥ 2.
General Strategies for Function Transformations
Understanding these two examples highlights several crucial strategies for handling any function transformation:
-
Order of Operations: Transformations are generally applied in the following order: horizontal shifts, stretches/compressions, reflections, and then vertical shifts. However, careful attention to the order within parentheses and exponentiation is crucial.
-
Parent Function Recognition: Identifying the parent function (e.g.,
x²,√x,|x|, etc.) is the first step. This helps visualize the original shape and how transformations alter it. -
Visualizing Transformations: Sketching graphs, even rough ones, can significantly improve understanding. Start with the parent function and apply each transformation sequentially.
-
Key Points: Focus on key features such as intercepts, vertices, and asymptotes. These points provide a solid framework for sketching the transformed function.
-
Domain and Range: Transformations can affect the domain and range of a function. Always consider how the transformations impact the allowable input and output values.
-
Practice Makes Perfect: The key to mastering function transformations is practice. Work through various problems, focusing on different types of functions and combinations of transformations.
Advanced Transformation Problems and Concepts
Once you've grasped the fundamentals, you can explore more complex scenarios:
-
Multiple Transformations: Problems might involve a sequence of multiple transformations, requiring careful consideration of their order and cumulative effects.
-
Piecewise Functions: Applying transformations to piecewise functions requires analyzing the effect on each piece of the function separately.
-
Inverse Functions: Transformations can also be applied to inverse functions, necessitating a deeper understanding of inverse relationships.
-
Trigonometric Functions: Transforming trigonometric functions introduces new concepts like phase shifts and amplitude changes.
Conclusion: Mastering Function Transformations
Function transformations are a vital topic in mathematics. By understanding the core principles – horizontal and vertical shifts, stretches and compressions, and reflections – you'll develop a powerful toolset for analyzing and manipulating functions. The key is consistent practice and a systematic approach to breaking down the transformation steps. This article provides a solid foundation, but continued practice and exploration of more challenging problems will solidify your understanding and ability to tackle any function transformation question with confidence. Remember to focus on understanding the why behind the transformations, not just the how. This deeper understanding will serve you well in advanced mathematical studies.
Latest Posts
Latest Posts
-
Select The Most Acidic Type Of Hydrogen In This Molecule
Apr 04, 2025
-
Managerial Accounting Information Is Normally Provided To Managers
Apr 04, 2025
-
What Are Two More Purposes Of The Violent Incident Log
Apr 04, 2025
-
Capital Budgeting Is Primarily Concerned With
Apr 04, 2025
-
Which Of The Following Statement Is False
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about 1-2 Additional Practice Transformations Of Functions Answers . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
