The Highest Barrier That A Projectile Can Clear Is
Holbox
Mar 28, 2025 · 5 min read
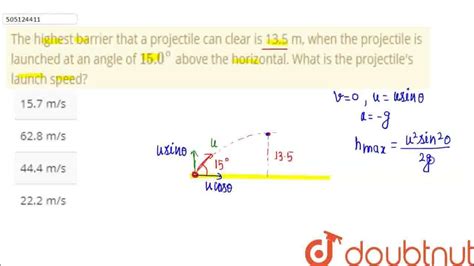
Table of Contents
- The Highest Barrier That A Projectile Can Clear Is
- Table of Contents
- The Highest Barrier a Projectile Can Clear: Exploring the Physics of Range and Trajectory
- Understanding Projectile Motion: The Fundamentals
- Horizontal Component: Constant Velocity
- Vertical Component: Accelerated Motion
- The Launch Angle: A Critical Parameter
- Maximum Height: Reaching the Apex
- Maximum Range: Optimizing Horizontal Distance
- The Interplay of Range and Height: Finding the Optimal Trajectory
- Introducing Air Resistance: A More Realistic Scenario
- Computational Methods and Simulation: Tackling Complexities
- Real-World Applications and Examples
- Conclusion: The Dynamic Nature of Projectile Motion
- Latest Posts
- Latest Posts
- Related Post
The Highest Barrier a Projectile Can Clear: Exploring the Physics of Range and Trajectory
The question of the highest barrier a projectile can clear is deceptively simple. While seemingly straightforward, it delves deep into the fascinating world of projectile motion, requiring a nuanced understanding of several key factors: initial velocity, launch angle, gravity, and air resistance. This exploration will unravel the complexities involved, revealing how these elements interplay to determine a projectile's maximum clearing height. We'll move beyond simple textbook examples and explore scenarios with more realistic considerations.
Understanding Projectile Motion: The Fundamentals
At its core, projectile motion is the curved path followed by an object launched into the air, subject only to the influence of gravity (and optionally, air resistance). Ignoring air resistance for the moment – a simplification frequently employed in introductory physics – the motion can be analyzed as two independent components: horizontal and vertical.
Horizontal Component: Constant Velocity
The horizontal velocity of a projectile remains constant throughout its flight, assuming no air resistance. This is because there are no horizontal forces acting upon it (ignoring air resistance). This constant velocity is simply the initial horizontal velocity, Vx, determined by the initial launch velocity and launch angle.
Vertical Component: Accelerated Motion
The vertical component, however, is governed by gravity, resulting in constant downward acceleration (approximately 9.8 m/s² on Earth). This acceleration affects the vertical velocity (Vy), continuously decreasing the upward velocity during the ascent and increasing the downward velocity during the descent.
The Launch Angle: A Critical Parameter
The launch angle, θ, significantly influences the projectile's trajectory. It determines the initial horizontal and vertical velocity components:
- Vx = V₀ cos θ
- Vy = V₀ sin θ
Where V₀ represents the initial launch velocity. Different launch angles result in different trajectories, optimizing for either maximum range or maximum height.
Maximum Height: Reaching the Apex
The highest point of a projectile's trajectory, its apex, occurs when the vertical velocity (Vy) becomes zero. At this moment, the projectile momentarily stops ascending before beginning its descent. We can use kinematic equations to determine this maximum height (H):
- Vy² = V₀y² + 2aΔy
Where:
- Vy = final vertical velocity (0 at the apex)
- V₀y = initial vertical velocity (V₀ sin θ)
- a = acceleration due to gravity (-g)
- Δy = vertical displacement (H)
Solving for H, we get:
- H = (V₀ sin θ)² / 2g
This equation demonstrates that the maximum height is directly proportional to the square of the initial velocity and the square of the sine of the launch angle. Maximum height is achieved when the launch angle is 90 degrees (straight upward), but this results in zero range.
Maximum Range: Optimizing Horizontal Distance
The maximum horizontal distance, or range (R), a projectile travels is achieved at a launch angle of 45 degrees (again, ignoring air resistance). This angle provides an optimal balance between horizontal and vertical velocities. The range equation is:
- R = (V₀² sin 2θ) / g
Note that the range is maximized when sin 2θ is maximized, which occurs at θ = 45 degrees. At this angle, sin 2θ = sin 90° = 1.
The Interplay of Range and Height: Finding the Optimal Trajectory
The problem of clearing the highest barrier involves finding the launch angle that maximizes the height at a given range, or vice versa. There isn't a single, universally applicable equation to solve this, as the optimal trajectory depends heavily on the barrier's height and distance.
For example, clearing a tall, close barrier might require a high launch angle, sacrificing range for height. Conversely, clearing a lower barrier located far away demands a flatter trajectory, maximizing range at the expense of height.
Introducing Air Resistance: A More Realistic Scenario
The previous analysis simplifies the real-world scenario by neglecting air resistance. Air resistance, or drag, is a force opposing the motion of the projectile, dependent on its velocity, shape, and the density of the air. Drag significantly complicates the analysis, as it's not a constant force but rather a function of the projectile's velocity. This means the horizontal velocity is no longer constant.
The effect of air resistance is twofold:
- Reduced Range: Drag diminishes both horizontal and vertical velocities, leading to a shorter range than predicted by the ideal model.
- Reduced Maximum Height: Drag opposes the upward motion, reducing the maximum height the projectile can reach.
- Asymmetrical Trajectory: The trajectory becomes asymmetrical, with a steeper descent than ascent.
Incorporating air resistance requires more complex mathematical models, often involving numerical solutions rather than closed-form equations. Factors such as the projectile's shape (drag coefficient), its mass, and the density of the air all play crucial roles.
Computational Methods and Simulation: Tackling Complexities
The complexities introduced by air resistance frequently necessitate computational methods and simulations. Software packages and programming languages (like Python with libraries such as NumPy and SciPy) can be used to model projectile motion, incorporating air resistance and other realistic factors. These simulations allow for accurate prediction of trajectories and the determination of the optimal launch parameters for clearing a given barrier.
Real-World Applications and Examples
The principles of projectile motion are applied across numerous fields:
- Sports: Baseball, basketball, golf, and many other sports rely on understanding projectile motion to optimize performance. The optimal launch angle and velocity for a long-distance shot, for example, depend heavily on the consideration of air resistance.
- Military Ballistics: The trajectory of artillery shells, missiles, and other projectiles is crucial in military applications. Accurate predictions demand complex models that account for various factors, including air density variations with altitude.
- Engineering: Designing systems that launch objects, from rockets to catapults, requires a thorough understanding of projectile motion and optimization techniques.
Conclusion: The Dynamic Nature of Projectile Motion
The question of the highest barrier a projectile can clear doesn't have a single, simple answer. It's a question that highlights the dynamic interplay of initial velocity, launch angle, gravity, and air resistance. While simplified models provide a useful starting point, a complete understanding necessitates acknowledging the complexity introduced by air resistance. Computational methods and simulations become indispensable tools for accurate prediction and optimization in real-world scenarios. The highest barrier depends not only on the projectile's capabilities but also on the careful consideration and optimization of its launch parameters, accounting for all relevant environmental factors. The quest to find the optimal solution is a continuing challenge in physics and engineering.
Latest Posts
Latest Posts
-
Which Of The Following May Indicate A Potential Drug Overdose
Mar 31, 2025
-
Internal Control Procedures For Cash Receipts Do Not Require That
Mar 31, 2025
-
A Is One Way Of Reducing Perceived Waiting Time
Mar 31, 2025
-
Theory And Practice Of Counseling And Psychotherapy
Mar 31, 2025
-
250 Lb Ft Over Turned To 320
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about The Highest Barrier That A Projectile Can Clear Is . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
