Select The Graph That Is Positively Skewed.
Holbox
Mar 31, 2025 · 6 min read
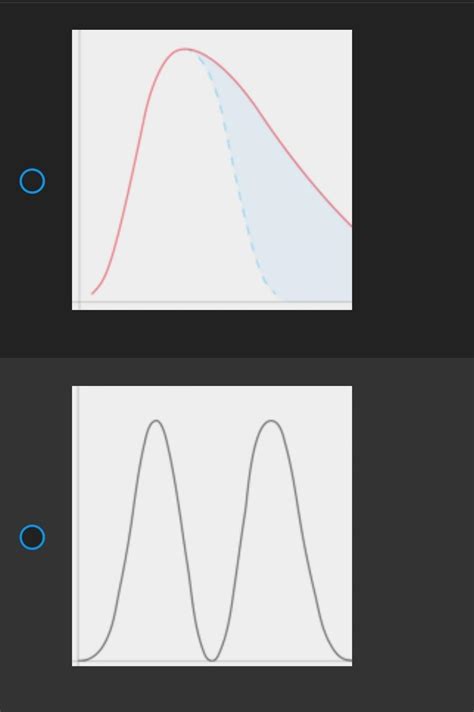
Table of Contents
- Select The Graph That Is Positively Skewed.
- Table of Contents
- Selecting the Positively Skewed Graph: A Comprehensive Guide
- What is Skewness?
- Identifying a Positively Skewed Graph
- 1. The Tail: The Defining Feature
- 2. Relationship Between Mean, Median, and Mode
- 3. Visual Inspection of Data Points Clustering
- 4. Comparing to Symmetrical and Negatively Skewed Distributions
- Examples of Positively Skewed Data
- 1. Income Distribution
- 2. House Prices
- 3. Exam Scores
- 4. Waiting Times in a Queue
- 5. Company Sizes (Number of Employees)
- Graphical Representations and Identification
- 1. Histograms
- 2. Box Plots
- 3. Frequency Polygons
- Practical Application: How to Determine Skewness in Your Data
- Conclusion: Mastering the Art of Identifying Positively Skewed Graphs
- Latest Posts
- Latest Posts
- Related Post
Selecting the Positively Skewed Graph: A Comprehensive Guide
Understanding data distribution is crucial in statistics and data analysis. One key aspect of understanding distribution is identifying skewness, which describes the asymmetry of a probability distribution. This article delves into the specifics of positive skewness, helping you confidently identify positively skewed graphs from various visual representations. We'll explore the characteristics of positive skewness, contrast it with other types of distributions, and provide practical examples to solidify your understanding.
What is Skewness?
Skewness is a measure of the asymmetry of the probability distribution of a real-valued random variable about its mean. In simpler terms, it tells us whether the tail of a distribution is longer on one side than the other. There are three main types of skewness:
-
Positive Skewness (Right Skewness): The tail on the right side of the distribution is longer or fatter. The mean is typically greater than the median, which is greater than the mode.
-
Negative Skewness (Left Skewness): The tail on the left side of the distribution is longer or fatter. The mean is typically less than the median, which is less than the mode.
-
Zero Skewness (Symmetrical): The distribution is perfectly symmetrical around the mean. The mean, median, and mode are all equal.
Identifying a Positively Skewed Graph
A positively skewed graph, also known as a right-skewed graph, displays several key characteristics that distinguish it from other distributions. Let's examine these visual cues:
1. The Tail: The Defining Feature
The most prominent feature of a positively skewed graph is its long right tail. This tail stretches out to the right, indicating a concentration of data points at lower values and a few outliers at much higher values. Imagine the graph as a hill; in a positively skewed distribution, the hill slopes gently down to the right, with a long, gradual descent.
2. Relationship Between Mean, Median, and Mode
In a positively skewed distribution, the relationship between the mean, median, and mode follows a specific order: Mean > Median > Mode. The mean is pulled to the right by the few high values in the tail, while the median and mode remain closer to the bulk of the data points. This order is a reliable indicator of positive skewness.
3. Visual Inspection of Data Points Clustering
Observe the clustering of data points. In a positively skewed graph, most data points will cluster towards the left side (lower values), with progressively fewer data points as you move towards the right (higher values). This creates the characteristic elongated tail on the right.
4. Comparing to Symmetrical and Negatively Skewed Distributions
To confidently identify a positively skewed graph, it's helpful to compare it to other distributions.
-
Symmetrical Distribution: A symmetrical distribution, like a normal distribution, is bell-shaped and perfectly balanced around the mean. The mean, median, and mode are equal. There's no long tail on either side.
-
Negatively Skewed Distribution: A negatively skewed distribution has a long tail on the left side. The mean is less than the median, which is less than the mode. The data points cluster towards the right, with fewer points towards the left tail.
Examples of Positively Skewed Data
Understanding real-world examples can greatly enhance your ability to recognize positive skewness. Here are some typical scenarios:
1. Income Distribution
Income data often exhibits positive skewness. The majority of people earn moderate incomes, while a small percentage of high-earners significantly inflate the mean. This creates a long right tail in the distribution.
2. House Prices
Similar to income, house prices frequently display positive skewness. Most houses fall within a certain price range, but a few luxury properties at significantly higher prices skew the distribution to the right.
3. Exam Scores
Exam scores can be positively skewed if the test is relatively easy. Many students achieve high scores, while a few students score much lower, resulting in a longer right tail.
4. Waiting Times in a Queue
Waiting times in a long queue often follow a positively skewed distribution. Most people wait for a relatively short time, but a few individuals might experience exceptionally long waits due to unforeseen circumstances.
5. Company Sizes (Number of Employees)
The distribution of company sizes (measured by the number of employees) often shows positive skewness. A large number of small companies exist, while fewer very large companies significantly impact the mean.
Graphical Representations and Identification
Positively skewed data can be visually represented using various graphs. Let’s consider how to identify positive skewness in different graphical representations:
1. Histograms
In a histogram, positive skewness is apparent through the longer tail extending to the right. The bars representing higher values are progressively shorter, while the bars representing lower values are taller and clustered. The mode (highest bar) will typically be to the left of the mean.
2. Box Plots
Box plots effectively illustrate skewness. In a positively skewed distribution, the median line will be closer to the bottom of the box, and the right whisker (representing the upper quartile and potential outliers) will be significantly longer than the left whisker. Outliers will tend to appear on the higher end of the scale.
3. Frequency Polygons
Frequency polygons, essentially line graphs connecting the midpoints of histogram bars, also exhibit the right-skewed nature through a longer right tail. The peak of the curve will be to the left of the mean.
Practical Application: How to Determine Skewness in Your Data
Now that we've explored the theoretical aspects, let's delve into the practical steps involved in identifying positive skewness in your own data set.
-
Data Collection and Organization: Gather your data and organize it efficiently. Spreadsheets or statistical software are invaluable tools.
-
Descriptive Statistics: Calculate the mean, median, and mode of your data. The relationship between these three measures will be a key indicator of skewness. If Mean > Median > Mode, it points towards positive skewness.
-
Visualization: Create a histogram, box plot, or frequency polygon of your data. Visually inspect the graph for a long right tail and the distribution of data points. This provides a strong visual confirmation of the skewness.
-
Statistical Measures of Skewness: Several statistical measures exist to quantify skewness, such as Pearson's moment coefficient of skewness and Bowley's skewness. These measures provide numerical values that confirm your visual assessment. However, visual inspection remains crucial for a complete understanding.
Conclusion: Mastering the Art of Identifying Positively Skewed Graphs
The ability to accurately identify positively skewed graphs is an essential skill in data analysis. By understanding the visual cues, the relationship between mean, median, and mode, and by utilizing appropriate graphical representations, you can confidently determine whether your data exhibits positive skewness. Remember that combining visual inspection with descriptive statistics and potentially statistical measures of skewness allows for a comprehensive understanding and avoids misinterpretations. Mastering this skill will empower you to make better informed decisions based on your data. Remember that positive skewness is simply one characteristic of data; understanding it in context with other statistical measures is key to a thorough analysis.
Latest Posts
Latest Posts
-
Art Labeling Activity Overview Of Cranial Nerves
Apr 03, 2025
-
Refer To The Graphic What Type Of Cabling Is Shown
Apr 03, 2025
-
Activity A Continued From Previous Page
Apr 03, 2025
-
What Three Joint Actions Comprise Triple Flexion During Sprinting
Apr 03, 2025
-
What Is The Conjugate Acid Of Hso4
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Select The Graph That Is Positively Skewed. . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
