Kirchhoff's Loop Rule Is A Statement Of
Holbox
Mar 29, 2025 · 6 min read
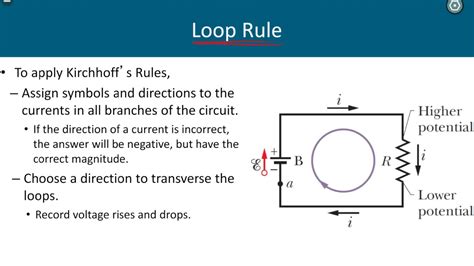
Table of Contents
- Kirchhoff's Loop Rule Is A Statement Of
- Table of Contents
- Kirchhoff's Loop Rule: A Statement of Conservation of Energy
- Understanding the Loop Rule: A Closed Path Journey
- Defining Potential Difference
- Applying the Loop Rule: A Step-by-Step Approach
- Examples Illustrating Kirchhoff's Loop Rule
- Example 1: A Simple Series Circuit
- Example 2: A Circuit with Multiple Loops and Branches
- Example 3: Circuits with Capacitors and Inductors
- Significance and Applications of Kirchhoff's Loop Rule
- Kirchhoff's Loop Rule and Other Conservation Laws
- Beyond the Basics: Advanced Applications
- Conclusion: A Cornerstone of Circuit Analysis
- Latest Posts
- Latest Posts
- Related Post
Kirchhoff's Loop Rule: A Statement of Conservation of Energy
Kirchhoff's loop rule, also known as Kirchhoff's voltage law (KVL), is a fundamental principle in circuit analysis. It's a powerful tool for understanding and predicting the behavior of complex electrical circuits. But what exactly is it a statement of? At its core, Kirchhoff's loop rule is a statement of the conservation of energy in electrical circuits. Let's delve deeper into this crucial concept, exploring its implications and applications.
Understanding the Loop Rule: A Closed Path Journey
Imagine tracing a path around a closed loop within an electrical circuit. This loop can be any continuous path that starts and ends at the same point, encompassing various components like resistors, capacitors, inductors, and voltage sources. Kirchhoff's loop rule states that the algebraic sum of the potential differences (voltages) around any closed loop in a circuit is always zero.
This seemingly simple statement has profound implications. It's a direct consequence of the conservative nature of the electrostatic field. The electric potential at a point is a scalar quantity, and the work done in moving a unit charge between two points is independent of the path taken. This means that if you complete a closed loop, the net work done on a charge is zero. Therefore, the total voltage drop (or rise) around the loop must also be zero.
Defining Potential Difference
Before proceeding, let's solidify our understanding of potential difference. Potential difference, or voltage, represents the energy per unit charge required to move a charge between two points in an electric field. A positive potential difference indicates a rise in potential, while a negative potential difference indicates a drop in potential. This is crucial for applying the loop rule correctly.
Applying the Loop Rule: A Step-by-Step Approach
Applying Kirchhoff's loop rule effectively involves a systematic approach:
-
Choose a Loop: Select a closed loop within the circuit. You can analyze multiple loops in a complex circuit.
-
Assign Current Directions: Arbitrarily assign a direction for the current in each branch of the loop. The direction doesn't need to be the actual direction; if your assumed direction is incorrect, the resulting current value will simply be negative.
-
Traverse the Loop: Start at any point in the chosen loop and traverse the loop in your chosen direction (clockwise or counterclockwise). As you move through each circuit element, account for the potential difference:
-
Resistor: If you traverse a resistor in the direction of the current, the potential difference is -IR (a voltage drop). If you traverse it against the current, the potential difference is +IR (a voltage rise). Here, 'I' represents the current and 'R' represents the resistance.
-
Voltage Source: If you traverse a voltage source from the negative terminal to the positive terminal, the potential difference is +V (a voltage rise). If you traverse it from positive to negative, the potential difference is -V (a voltage drop).
-
-
Sum the Potential Differences: Add up all the potential differences encountered while traversing the loop. Remember to account for the sign (+ or -) of each potential difference.
-
Set the Sum to Zero: According to Kirchhoff's loop rule, the algebraic sum of these potential differences must equal zero: ΣV = 0.
Examples Illustrating Kirchhoff's Loop Rule
Let's illustrate the application of Kirchhoff's loop rule with a few examples.
Example 1: A Simple Series Circuit
Consider a simple series circuit with a battery (voltage source, V) and a resistor (R). Applying the loop rule:
- Traverse the loop starting from the negative terminal of the battery.
- We encounter a voltage rise of +V across the battery.
- We then traverse the resistor in the direction of the current, encountering a voltage drop of -IR.
- According to Kirchhoff's loop rule: +V - IR = 0. This equation directly gives us Ohm's Law: V = IR.
Example 2: A Circuit with Multiple Loops and Branches
A more complex circuit might involve multiple loops and branches, requiring the application of both Kirchhoff's loop rule and Kirchhoff's junction rule (which deals with current conservation at junctions). To solve such circuits, you'll need to write a system of equations based on the loop rule for each loop and the junction rule for each junction. Solving this system of equations will provide the currents in each branch of the circuit.
Example 3: Circuits with Capacitors and Inductors
Kirchhoff's loop rule can also be applied to circuits containing capacitors and inductors, but the equations become more complex. For capacitors, the potential difference is given by V = Q/C (where Q is the charge and C is the capacitance), and for inductors, the potential difference is given by V = L(dI/dt) (where L is the inductance and dI/dt is the rate of change of current). These equations must be incorporated into the loop equations.
Significance and Applications of Kirchhoff's Loop Rule
The loop rule is an indispensable tool in circuit analysis, with wide-ranging applications:
-
Circuit Design: Engineers use Kirchhoff's laws extensively in designing electronic circuits to ensure proper voltage and current levels.
-
Fault Diagnosis: Troubleshooting faulty circuits often involves applying Kirchhoff's laws to identify the location of faults.
-
Power Systems Analysis: Kirchhoff's laws are critical in analyzing power distribution networks to ensure efficient power delivery and prevent overloads.
-
Electronics and Telecommunications: From simple household appliances to complex communication systems, Kirchhoff's laws form the foundation of circuit analysis and design.
Kirchhoff's Loop Rule and Other Conservation Laws
The loop rule is fundamentally a manifestation of the conservation of energy. The total energy supplied by the sources in a closed loop must equal the total energy dissipated or stored in the circuit elements. No energy is created or destroyed; it simply transforms from one form to another.
This ties in with other fundamental conservation laws in physics:
-
Conservation of Charge: Kirchhoff's junction rule is a statement of the conservation of charge, ensuring that the current entering a junction equals the current leaving it.
-
Conservation of Momentum: While not directly related to the loop rule, the concept of momentum conservation also underlies many physical phenomena, including the movement of charged particles in electric and magnetic fields.
Beyond the Basics: Advanced Applications
The loop rule, while seemingly simple, becomes significantly more complex when dealing with:
-
Non-linear circuits: Circuits with components whose behavior isn't linearly proportional to voltage or current.
-
Time-varying circuits: Circuits where voltages and currents change with time.
-
AC circuits: Circuits using alternating current, requiring the use of phasor analysis.
Conclusion: A Cornerstone of Circuit Analysis
Kirchhoff's loop rule, a direct consequence of energy conservation, stands as a cornerstone of circuit analysis. Its straightforward application in simple circuits and its adaptable nature in more complex scenarios make it an invaluable tool for anyone studying or working with electrical circuits. Understanding its implications and mastering its application is essential for anyone seeking a solid grasp of electrical engineering principles. By consistently applying this rule and combining it with other analytical techniques, we can accurately predict and analyze the behavior of even the most intricate electrical systems. The enduring relevance of Kirchhoff’s loop rule underscores its importance as a fundamental principle in the field of electrical engineering and beyond. It’s a testament to the power of fundamental physical laws in providing a robust framework for understanding complex systems.
Latest Posts
Latest Posts
-
The Different Dimensions Within Leadership Ethics Influence One Another
Apr 01, 2025
-
What Does Being A Manager Offer To An Employee
Apr 01, 2025
-
The Function Requires That Management Evaluate Operations Against Some Norm
Apr 01, 2025
-
Select The Two Primary Characteristics That Define Advertising
Apr 01, 2025
-
Trade Can Make Everyone Better Off Because It
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Kirchhoff's Loop Rule Is A Statement Of . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
