How Should You Arrange Conversion Factors In Dimensional Analysis
Holbox
Mar 29, 2025 · 5 min read
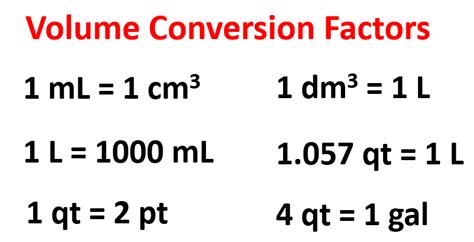
Table of Contents
- How Should You Arrange Conversion Factors In Dimensional Analysis
- Table of Contents
- Mastering Dimensional Analysis: A Comprehensive Guide to Arranging Conversion Factors
- Understanding Conversion Factors
- The Strategy: Cancelling Units
- Multiple Conversions: A Step-by-Step Approach
- Dealing with Exponents and Complex Units
- Common Pitfalls and How to Avoid Them
- Advanced Applications and Problem-Solving Strategies
- Conclusion: Mastering the Art of Conversion Factors
- Latest Posts
- Latest Posts
- Related Post
Mastering Dimensional Analysis: A Comprehensive Guide to Arranging Conversion Factors
Dimensional analysis, also known as the factor-label method, is a powerful problem-solving technique used extensively in science and engineering. It allows us to convert units and solve complex problems by focusing on the relationships between different units of measurement. The key to successful dimensional analysis lies in the skillful arrangement of conversion factors. This article provides a comprehensive guide to mastering this crucial aspect, equipping you with the knowledge and confidence to tackle any unit conversion challenge.
Understanding Conversion Factors
At the heart of dimensional analysis lies the conversion factor. A conversion factor is a fraction where the numerator and denominator represent the same quantity but in different units. Because the numerator and denominator are equivalent, the conversion factor is essentially equal to 1. Multiplying a quantity by a conversion factor does not change its value, only its units.
For example, consider converting inches to centimeters. We know that 1 inch is equal to 2.54 centimeters. Therefore, we can create two conversion factors:
- 1 inch / 2.54 cm (Used when we want to cancel out inches)
- 2.54 cm / 1 inch (Used when we want to cancel out centimeters)
The choice of which conversion factor to use depends entirely on the units you're starting with and the units you want to end up with. This is where careful arrangement comes into play.
The Strategy: Cancelling Units
The core principle of arranging conversion factors is unit cancellation. We strategically choose conversion factors to ensure that unwanted units cancel out, leaving us with the desired units. Imagine units as algebraic variables; we can cancel them in the same way we cancel variables in algebraic expressions.
Let's illustrate this with an example: Convert 15 inches to meters.
We know the following:
- 1 inch = 2.54 cm
- 100 cm = 1 meter
We need to arrange the conversion factors so that "inches" and "centimeters" cancel out, leaving us with "meters".
15 inches * (2.54 cm / 1 inch) * (1 meter / 100 cm) = 0.381 meters
Notice how "inches" cancels with "inches" and "centimeters" cancels with "centimeters," leaving only "meters" in the final answer.
Key takeaway: Always write out your units and cancel them systematically. This is the single most important step to avoiding errors in dimensional analysis.
Multiple Conversions: A Step-by-Step Approach
Many problems require multiple conversion factors. The process remains the same; we simply chain together multiple conversion factors to reach the desired units. The key is to carefully plan the sequence of conversions.
Example: Convert 60 miles per hour to meters per second.
We'll need several conversion factors:
- 1 mile = 5280 feet
- 1 foot = 12 inches
- 1 inch = 2.54 cm
- 100 cm = 1 meter
- 1 hour = 60 minutes
- 1 minute = 60 seconds
Let's arrange them carefully:
60 miles/hour * (5280 feet/1 mile) * (12 inches/1 foot) * (2.54 cm/1 inch) * (1 meter/100 cm) * (1 hour/60 minutes) * (1 minute/60 seconds) = 26.82 meters/second
Observe how the units cancel out systematically, leading us to the final answer in meters per second.
Dealing with Exponents and Complex Units
Conversion factors also work seamlessly with units raised to powers (e.g., square meters, cubic centimeters) and complex units involving multiple dimensions (e.g., density, velocity). The key is to apply the conversion factors to each component of the unit individually.
Example: Convert 10 cubic feet to cubic meters.
Since 1 foot = 0.3048 meters, we cube the conversion factor:
10 ft³ * (0.3048 m/1 ft)³ = 0.283 m³
Notice how we cubed the conversion factor to account for the cubic unit.
Example involving multiple units: Convert a density of 2.7 g/cm³ to kg/m³.
We'll need conversion factors for both grams to kilograms and cubic centimeters to cubic meters:
2.7 g/cm³ * (1 kg/1000 g) * (100 cm/1 m)³ = 2700 kg/m³
Again, we carefully arrange the conversion factors to cancel out the unwanted units. The cubic conversion factor is crucial here.
Common Pitfalls and How to Avoid Them
Despite its simplicity, dimensional analysis can be prone to errors. Here are some common pitfalls and how to avoid them:
-
Incorrect Conversion Factor Arrangement: Always double-check that your conversion factors are arranged correctly to ensure proper unit cancellation. Writing out the units explicitly helps tremendously.
-
Forgetting to Cube or Square Units: When dealing with squared or cubed units, remember to raise the conversion factor to the corresponding power.
-
Mixing Up Units: Pay close attention to unit prefixes (e.g., kilo, milli, micro) and ensure consistency throughout your calculations.
-
Mathematical Errors: While dimensional analysis focuses on units, it's still essential to perform the mathematical operations correctly. Use a calculator if necessary and double-check your work.
Advanced Applications and Problem-Solving Strategies
Dimensional analysis isn't just for simple unit conversions; it's a versatile tool with broader applications:
-
Checking the Validity of Equations: Dimensional analysis can be used to verify whether a physics equation is dimensionally consistent. Both sides of the equation must have the same units.
-
Deriving Equations: While not directly deriving equations, dimensional analysis can provide hints about the form an equation might take.
-
Estimating Quantities: Dimensional analysis can be used to make quick estimates of quantities, even if you lack precise values.
General problem-solving strategy:
- Identify the starting units and the desired units.
- Find the appropriate conversion factors. Consult reference tables or textbooks as needed.
- Carefully arrange the conversion factors to cancel unwanted units. Visualize the unit cancellation process.
- Perform the mathematical calculations. Use a calculator if necessary.
- Check your answer: Make sure the units are correct and the numerical value is reasonable.
Conclusion: Mastering the Art of Conversion Factors
Mastering dimensional analysis and the skillful arrangement of conversion factors is crucial for success in scientific and engineering endeavors. By focusing on unit cancellation and systematically applying conversion factors, you can confidently solve even the most complex unit conversion problems. Remember the importance of meticulous attention to detail, careful planning of the conversion sequence, and consistent unit tracking. With practice and a systematic approach, dimensional analysis becomes an invaluable problem-solving tool. Embrace the power of unit cancellation, and you'll unlock a whole new level of confidence and efficiency in your scientific and engineering calculations.
Latest Posts
Latest Posts
-
Major Convictions Will Affect Eligibility Under What Criteria
Apr 02, 2025
-
To Develop Psychographic Segments The Marketer Must Understand Consumers
Apr 02, 2025
-
Two Parallel Lines Cut By A Transversal Answer Key
Apr 02, 2025
-
Which Of The Following Is Associated With Port Security
Apr 02, 2025
-
What Does A Dead Battery Mean Chemically
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about How Should You Arrange Conversion Factors In Dimensional Analysis . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
