Evaluate The Integral By Changing To Cylindrical Coordinates
Holbox
Mar 28, 2025 · 6 min read
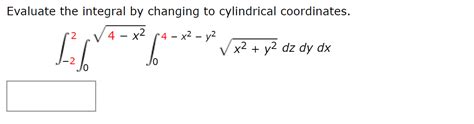
Table of Contents
- Evaluate The Integral By Changing To Cylindrical Coordinates
- Table of Contents
- Evaluating Integrals by Changing to Cylindrical Coordinates: A Comprehensive Guide
- Understanding Cylindrical Coordinates
- The Jacobian Determinant: A Crucial Component
- Step-by-Step Guide to Evaluating Triple Integrals in Cylindrical Coordinates
- Illustrative Examples
- Common Mistakes to Avoid
- Conclusion
- Latest Posts
- Latest Posts
- Related Post
Evaluating Integrals by Changing to Cylindrical Coordinates: A Comprehensive Guide
Switching to cylindrical coordinates is a powerful technique in multivariable calculus for simplifying the evaluation of triple integrals, particularly those involving regions with cylindrical symmetry. This comprehensive guide will delve into the intricacies of this method, providing a step-by-step approach with illustrative examples. We'll cover the core concepts, the transformation process, Jacobian determinants, and common pitfalls to avoid.
Understanding Cylindrical Coordinates
Before diving into the integration process, let's refresh our understanding of cylindrical coordinates. They offer a natural representation for regions that exhibit cylindrical symmetry. Instead of using the Cartesian coordinates (x, y, z), we employ (r, θ, z), where:
- r: The distance from the z-axis to the point (x, y, z) in the xy-plane. Note that r ≥ 0.
- θ: The angle between the positive x-axis and the line segment connecting the origin to the projection of (x, y, z) onto the xy-plane. The angle θ is measured counter-clockwise and typically ranges from 0 to 2π.
- z: The same z-coordinate as in the Cartesian system, representing the height.
The relationships between Cartesian and cylindrical coordinates are given by:
- x = r cos θ
- y = r sin θ
- z = z
These equations are crucial for transforming the integrand and the limits of integration.
The Jacobian Determinant: A Crucial Component
When changing variables in a multiple integral, the Jacobian determinant accounts for the scaling effect of the transformation. It ensures the integral remains accurate after the coordinate change. For cylindrical coordinates, the Jacobian is:
|J| = ∂(x, y, z) / ∂(r, θ, z) = r
This means that the differential volume element dV transforms as follows:
dV = dx dy dz = r dr dθ dz
The appearance of 'r' in the Jacobian is critical; omitting it is a common mistake leading to incorrect results. This factor adjusts the volume element to reflect the changing area of the infinitesimal cylindrical shell as 'r' varies.
Step-by-Step Guide to Evaluating Triple Integrals in Cylindrical Coordinates
Let's break down the process into manageable steps:
-
Identify the Region of Integration: Carefully examine the region of integration in the given problem. Is it a cylinder, a portion of a cylinder, or a region with cylindrical symmetry? Sketching the region is highly recommended. This visualization helps determine the limits of integration in cylindrical coordinates.
-
Transform the Integrand: Replace x, y, and z in the integrand with their cylindrical equivalents: x = r cos θ, y = r sin θ, and z = z. This step transforms the function from Cartesian to cylindrical representation.
-
Determine the Limits of Integration: This is often the most challenging step. Based on the geometry of the region, express the limits of integration for r, θ, and z. Remember that the limits must be consistent and reflect the boundaries of the region accurately. For example:
- r: This usually ranges from 0 to the radius of the cylinder or a function of θ.
- θ: This typically goes from 0 to 2π for full cylinders, but can be restricted to a smaller interval depending on the region.
- z: This might be constant limits or functions of r and θ, determined by the region's boundaries.
-
Apply the Jacobian Determinant: Remember to include the Jacobian determinant (r) in the integrand. This is the essential factor that corrects for the area scaling during the transformation.
-
Evaluate the Iterated Integral: Now that everything is in cylindrical coordinates, evaluate the resulting iterated integral. The order of integration (dr, dθ, dz or any other permutation) might affect the complexity of the calculation; strategically choosing the order can sometimes simplify the problem.
Illustrative Examples
Let's work through a few examples to solidify our understanding:
Example 1: Simple Cylinder
Evaluate the triple integral ∫∫∫<sub>E</sub> x² + y² dV, where E is the region inside the cylinder x² + y² = 9 and between the planes z = 0 and z = 5.
Solution:
- Region: E is a cylinder of radius 3 and height 5.
- Integrand: x² + y² = r²
- Limits: 0 ≤ r ≤ 3, 0 ≤ θ ≤ 2π, 0 ≤ z ≤ 5
- Integral: ∫<sub>0</sub><sup>5</sup> ∫<sub>0</sub><sup>2π</sup> ∫<sub>0</sub><sup>3</sup> r²(r dr dθ dz) = ∫<sub>0</sub><sup>5</sup> ∫<sub>0</sub><sup>2π</sup> [r⁴/4]<sub>0</sub><sup>3</sup> dθ dz = 81π(5) = 405π
Example 2: Region with Variable Limits
Evaluate ∫∫∫<sub>E</sub> z dV, where E is bounded by the paraboloid z = x² + y² and the plane z = 4.
Solution:
- Region: E is bounded above by the plane z = 4 and below by the paraboloid z = r².
- Integrand: z
- Limits: 0 ≤ r ≤ 2, 0 ≤ θ ≤ 2π, r² ≤ z ≤ 4
- Integral: ∫<sub>0</sub><sup>2π</sup> ∫<sub>0</sub><sup>2</sup> ∫<sub>r²</sub><sup>4</sup> z(r dz dr dθ) = ∫<sub>0</sub><sup>2π</sup> ∫<sub>0</sub><sup>2</sup> [(z²/2)r]<sub>r²</sub><sup>4</sup> dr dθ = ∫<sub>0</sub><sup>2π</sup> ∫<sub>0</sub><sup>2</sup> (8r - r⁵/2) dr dθ = 16π
Example 3: More Complex Region
Let's consider a region E defined by x² + y² ≤ 4, x ≥ 0, y ≥ 0, and 0 ≤ z ≤ 1. Evaluate ∫∫∫<sub>E</sub> xy dV.
Solution:
- Region: This represents a quarter-cylinder of radius 2 and height 1 in the first octant.
- Integrand: xy = (r cos θ)(r sin θ) = r² sin θ cos θ = (1/2)r² sin(2θ)
- Limits: 0 ≤ r ≤ 2, 0 ≤ θ ≤ π/2, 0 ≤ z ≤ 1
- Integral: ∫<sub>0</sub><sup>1</sup> ∫<sub>0</sub><sup>π/2</sup> ∫<sub>0</sub><sup>2</sup> (1/2)r³ sin(2θ) dr dθ dz = 2
Common Mistakes to Avoid
- Forgetting the Jacobian: The most frequent error is omitting the 'r' from the Jacobian determinant. This leads to an incorrect result.
- Incorrect Limits of Integration: Carefully determine the limits of integration based on the region's geometry. A mistake in the limits propagates to the final answer.
- Order of Integration: The order of integration can affect the difficulty of the calculation. Consider strategically choosing the order to simplify the integration process.
- Trigonometric Identities: Skillfully applying trigonometric identities can often simplify the integrand before integrating.
Conclusion
Changing to cylindrical coordinates significantly simplifies many triple integral problems, especially those involving regions with cylindrical symmetry. By carefully following the steps outlined above, including the correct application of the Jacobian determinant and the careful determination of limits of integration, you can efficiently and accurately solve these complex integration problems. Remember to visualize the region, carefully transform the integrand and limits, and always include the Jacobian to ensure your solution is correct. Practice is key to mastering this powerful technique.
Latest Posts
Latest Posts
-
Which Bacteria Cause The Greatest Harm In The Food Industry
Mar 31, 2025
-
Label The Specific Bony Features Of The Superior Skull
Mar 31, 2025
-
Draw The Major Elimination Product Formed In The Reaction
Mar 31, 2025
-
Identify The Indentation That Is Inferiorolateral To The Auricular Surface
Mar 31, 2025
-
Label The Parts Of An Earthquake
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Evaluate The Integral By Changing To Cylindrical Coordinates . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
