Evaluate The Integral. 1 0 12 4x2 5x 1 Dx
Holbox
May 10, 2025 · 4 min read
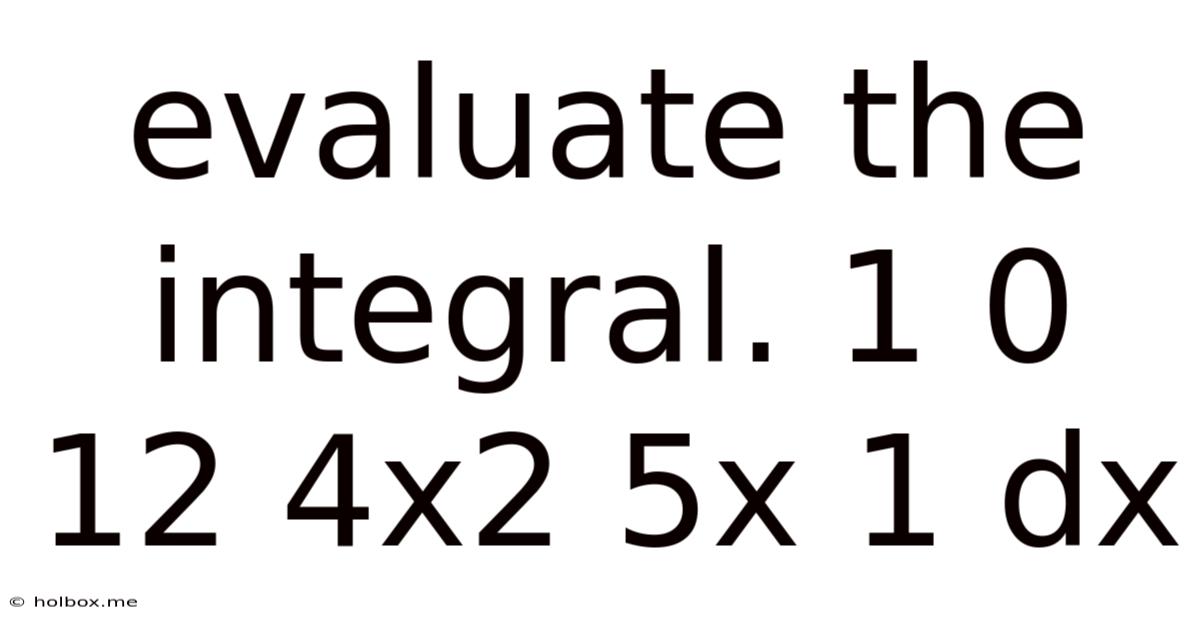
Table of Contents
- Evaluate The Integral. 1 0 12 4x2 5x 1 Dx
- Table of Contents
- Evaluating the Definite Integral: ∫₀¹ (12 + 4x² + 5x + 1) dx
- Understanding Definite Integrals
- Step-by-Step Solution
- 1. Simplify the Integrand
- 2. Apply the Power Rule of Integration
- 3. Combine the Integrated Terms
- 4. Evaluate the Definite Integral
- 5. Simplify the Result
- Graphical Interpretation
- Applications of Definite Integrals
- Extending the Concept
- Conclusion
- Latest Posts
- Related Post
Evaluating the Definite Integral: ∫₀¹ (12 + 4x² + 5x + 1) dx
This article provides a comprehensive guide on how to evaluate the definite integral ∫₀¹ (12 + 4x² + 5x + 1) dx. We'll break down the process step-by-step, explaining the underlying principles of integration and demonstrating the solution using both analytical methods and a conceptual approach to solidify understanding. We'll also touch upon practical applications and potential extensions of this type of problem.
Understanding Definite Integrals
Before diving into the specifics of this integral, let's establish a firm understanding of what a definite integral represents. In essence, a definite integral calculates the signed area between a function's curve and the x-axis within a specified interval. The "signed" aspect means that areas below the x-axis are considered negative, while areas above are positive. The definite integral is represented as:
∫ₐᵇ f(x) dx
where:
ais the lower limit of integration.bis the upper limit of integration.f(x)is the function being integrated.
In our case, a = 0, b = 1, and f(x) = 12 + 4x² + 5x + 1.
Step-by-Step Solution
To evaluate the definite integral ∫₀¹ (12 + 4x² + 5x + 1) dx, we'll follow these steps:
1. Simplify the Integrand
First, simplify the function inside the integral:
12 + 4x² + 5x + 1 = 13 + 4x² + 5x
Our integral now becomes:
∫₀¹ (13 + 4x² + 5x) dx
2. Apply the Power Rule of Integration
The power rule of integration states that:
∫xⁿ dx = (xⁿ⁺¹)/(n+1) + C
where 'n' is any real number except -1, and 'C' is the constant of integration. We'll apply this rule to each term in our integrand:
- ∫13 dx = 13x
- ∫4x² dx = (4x³)/3
- ∫5x dx = (5x²)/2
3. Combine the Integrated Terms
Combining the integrated terms, we get:
13x + (4x³)/3 + (5x²)/2
4. Evaluate the Definite Integral
Now we evaluate the definite integral by substituting the upper limit (1) and lower limit (0) into the result from step 3 and subtracting:
[13(1) + (4(1)³)/3 + (5(1)²)/2] - [13(0) + (4(0)³)/3 + (5(0)²)/2]
This simplifies to:
13 + (4/3) + (5/2) - 0
5. Simplify the Result
To simplify the result, find a common denominator for the fractions:
13 + (8/6) + (15/6) = 13 + (23/6)
Converting 13 to a fraction with a denominator of 6:
(78/6) + (23/6) = 101/6
Therefore, the value of the definite integral ∫₀¹ (12 + 4x² + 5x + 1) dx is 101/6 or approximately 16.833.
Graphical Interpretation
The definite integral represents the area under the curve of the function y = 13 + 4x² + 5x between x = 0 and x = 1. Visualizing this area helps solidify the meaning of the calculated value. While we can't create a visual graph here, imagine a parabola opening upwards, shifted upward and to the left – that's the shape of our function. The area between this parabola and the x-axis, from x=0 to x=1, is precisely 101/6 square units.
Applications of Definite Integrals
Definite integrals have numerous applications across various fields. Some notable examples include:
- Calculating areas: As demonstrated above, it's a fundamental tool for determining areas of irregular shapes bounded by curves.
- Determining volumes: Definite integrals extend to calculating volumes of solids of revolution.
- Physics: Calculating work done by a force, displacement, and other physical quantities.
- Engineering: Solving problems related to stress, strain, and other mechanical properties.
- Economics: Evaluating total cost, revenue, and profit functions.
- Probability and Statistics: Determining probabilities and expected values.
Extending the Concept
The problem we solved is a relatively straightforward example. More complex definite integrals might involve:
- Trigonometric functions: Integrals involving sin(x), cos(x), tan(x), etc., often require trigonometric identities and substitution techniques.
- Exponential and logarithmic functions: These functions require specific integration rules.
- Improper integrals: Integrals with infinite limits of integration or integrands with discontinuities within the integration interval.
- Integration by parts: A technique used to integrate products of functions.
- Partial fraction decomposition: A method for integrating rational functions.
Mastering these advanced techniques opens doors to solving a vast array of more challenging integration problems.
Conclusion
Evaluating definite integrals is a crucial skill in calculus and has far-reaching implications in diverse fields. This article has provided a detailed walkthrough of solving a specific definite integral, along with an explanation of the underlying concepts and potential extensions. Understanding the fundamentals of integration and applying the appropriate techniques are vital for solving more complex problems and leveraging the power of calculus in various applications. Remember that practice is key; the more you work through different integration problems, the more proficient you'll become.
Latest Posts
Related Post
Thank you for visiting our website which covers about Evaluate The Integral. 1 0 12 4x2 5x 1 Dx . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.