A Wave Having A Frequency Of 1000 Hertz Vibrates At
Holbox
Mar 29, 2025 · 6 min read
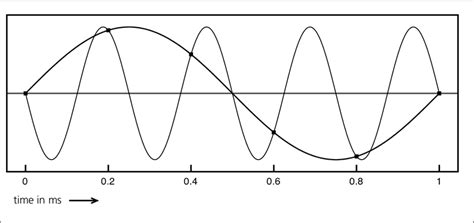
Table of Contents
- A Wave Having A Frequency Of 1000 Hertz Vibrates At
- Table of Contents
- A Wave Having a Frequency of 1000 Hertz Vibrates At... Exploring the World of Sound and Waves
- Understanding Hertz and Frequency
- The Audible Spectrum and 1000 Hz
- Wavelength and the Speed of Sound
- The Nature of Sound Waves
- Amplitude and Intensity
- Applications of 1000 Hz Waves
- Audio Engineering and Music
- Medical Applications
- Communications and Signal Processing
- Beyond the Basics: Interference and Resonance
- Interference
- Resonance
- Factors Affecting Wave Propagation
- Conclusion: The Significance of 1000 Hz and Beyond
- Latest Posts
- Latest Posts
- Related Post
A Wave Having a Frequency of 1000 Hertz Vibrates At... Exploring the World of Sound and Waves
A wave with a frequency of 1000 Hertz vibrates at 1000 cycles per second. This seemingly simple statement opens the door to a fascinating exploration of the world of sound, physics, and the properties of waves. Understanding this fundamental concept allows us to delve into various aspects of wave behavior, from the perception of sound by the human ear to the applications of this frequency in technology. This article will explore the implications of a 1000 Hz wave, its properties, and its relevance across different fields.
Understanding Hertz and Frequency
Before we delve deeper, let's solidify our understanding of the key term: Hertz (Hz). Hertz is the unit of frequency, representing the number of cycles or oscillations a wave completes in one second. In simpler terms, a wave with a frequency of 1000 Hz completes 1000 cycles—meaning a complete back-and-forth movement—in a single second. This frequency is within the audible range for humans, meaning we can hear it as a sound.
The Audible Spectrum and 1000 Hz
The human ear can typically perceive sounds within a frequency range of approximately 20 Hz to 20,000 Hz. A 1000 Hz wave falls comfortably within this range, placing it in the mid-range of human hearing. Sounds at this frequency are generally perceived as relatively clear and distinct. The pitch of a sound is directly related to its frequency; higher frequencies correspond to higher pitches, and lower frequencies correspond to lower pitches. A 1000 Hz tone would be perceived as a relatively high-pitched sound, though not as high as some sounds we can hear.
Wavelength and the Speed of Sound
The frequency of a wave is intrinsically linked to its wavelength and the speed of sound. Wavelength is the distance between two consecutive crests (or troughs) of a wave. The relationship between frequency (f), wavelength (λ), and the speed of sound (v) is given by the equation: v = fλ.
The speed of sound varies depending on the medium through which it travels. In dry air at 20°C (68°F), the speed of sound is approximately 343 meters per second (m/s). Therefore, for a 1000 Hz wave in this medium, the wavelength can be calculated:
λ = v/f = 343 m/s / 1000 Hz = 0.343 meters (approximately 34.3 centimeters)
The Nature of Sound Waves
Sound waves are longitudinal waves, meaning the particles of the medium (like air molecules) vibrate parallel to the direction of wave propagation. When a 1000 Hz wave travels through the air, air molecules compress and rarefy (spread out) rhythmically, creating regions of high and low pressure that propagate outwards. These pressure variations are what our ears detect and interpret as sound.
Amplitude and Intensity
The amplitude of a wave refers to its maximum displacement from its equilibrium position. In the context of sound, amplitude is directly related to the intensity or loudness of the sound. A higher amplitude corresponds to a louder sound, while a lower amplitude corresponds to a quieter sound. The intensity of a sound is usually measured in decibels (dB). A 1000 Hz wave can have varying amplitudes, resulting in sounds of different loudness.
Applications of 1000 Hz Waves
The 1000 Hz frequency, being within the audible range and having a readily calculable wavelength, finds applications in various fields:
Audio Engineering and Music
In audio engineering and music production, a thorough understanding of frequencies like 1000 Hz is crucial. This frequency often falls within the mid-range of many instruments and vocals, contributing significantly to the overall timbre and clarity of the sound. Equalization (EQ) techniques are used to adjust the levels of different frequencies, often focusing on the mid-range frequencies, including 1000 Hz, to achieve a balanced and pleasing sound.
Medical Applications
Certain medical imaging and therapeutic techniques utilize sound waves within the audible range, including frequencies around 1000 Hz. While not directly employing 1000 Hz specifically, the principles of wave propagation and frequency manipulation are central to these applications.
Communications and Signal Processing
Frequencies within the range of 1000 Hz are often used in communication systems, albeit usually modulated and combined with other frequencies. Signal processing techniques leverage frequency analysis to isolate and enhance desired signals while filtering out noise. The understanding of frequencies, their interaction, and propagation form the base for these advanced processes.
Beyond the Basics: Interference and Resonance
The behavior of a 1000 Hz wave is further influenced by phenomena like interference and resonance.
Interference
When two or more waves overlap, they interfere with each other. Constructive interference occurs when the waves are in phase, resulting in a wave with a larger amplitude. Destructive interference occurs when the waves are out of phase, resulting in a wave with a smaller amplitude or even cancellation. The effects of interference can significantly affect the perceived sound, leading to variations in loudness and tonal quality.
Resonance
Resonance occurs when an object vibrates at its natural frequency, leading to a significant increase in amplitude. This phenomenon is widely observed in musical instruments, where the instrument's design and material properties determine its resonant frequencies. A 1000 Hz wave could induce resonance in certain objects if their natural frequency is close to 1000 Hz.
Factors Affecting Wave Propagation
The propagation of a 1000 Hz wave is affected by several factors:
- Medium: The speed and attenuation (reduction in amplitude) of the wave depend on the properties of the medium it travels through (e.g., air, water, solids).
- Temperature: Temperature influences the speed of sound in gases.
- Humidity: Humidity affects the density of air and can slightly alter the speed of sound.
- Obstacles: Obstacles in the path of the wave can cause reflection, diffraction, and scattering.
Conclusion: The Significance of 1000 Hz and Beyond
A wave vibrating at 1000 Hz is more than just a simple physical phenomenon; it represents a fundamental concept in physics with wide-ranging implications. Understanding its properties – frequency, wavelength, amplitude, and interactions with the environment – provides a foundation for exploring the world of sound, its perception, and its applications across various fields. From the creation of music to advanced medical technologies and communication systems, the principles governing the behavior of waves like the 1000 Hz example are crucial to technological advancements and our understanding of the physical world. Further exploration into the intricacies of wave behavior and frequency manipulation will undoubtedly continue to shape innovation and technological progress in the years to come. This detailed analysis highlights the importance of understanding basic physics concepts and their profound influence on the technology we use daily.
Latest Posts
Latest Posts
-
You Receive An Email Marked Important From Your Boss
Apr 02, 2025
-
The Bureau Of Transportation Statistics Collects Analyzes And Disseminates
Apr 02, 2025
-
Stirring The Mixture Does Which Of The Following Select Two
Apr 02, 2025
-
Comity Is A Doctrine That Is Rooted In
Apr 02, 2025
-
What Material Makes Up Most Of The Structure At A
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about A Wave Having A Frequency Of 1000 Hertz Vibrates At . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
