A Block Is Resting On A Wooden Plank
Holbox
Mar 30, 2025 · 7 min read
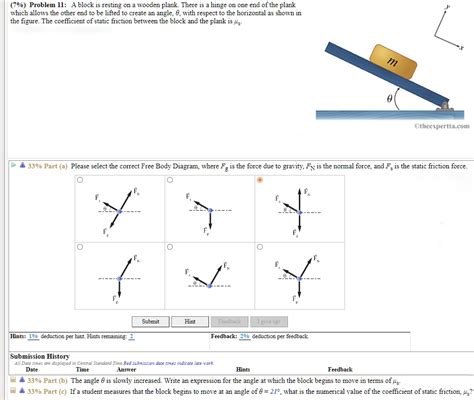
Table of Contents
- A Block Is Resting On A Wooden Plank
- Table of Contents
- A Block Resting on a Wooden Plank: A Deep Dive into Statics and Dynamics
- Static Equilibrium: The Foundation of Stability
- 1. Gravity: The Ever-Present Force
- 2. Normal Force: The Plank's Resistance
- 3. Friction: The Unsung Hero (and Villain)
- Factors Influencing Stability
- 1. The Coefficient of Friction: A Crucial Parameter
- 2. The Angle of Inclination: Tipping Point
- 3. The Block's Shape and Size: Center of Gravity Matters
- 4. The Plank's Properties: Rigidity and Surface
- Dynamic Considerations: Motion and Acceleration
- 1. Newton's Second Law: F = ma
- 2. Acceleration Down an Incline: Calculating Motion
- 3. Factors Affecting Acceleration: Friction and Inclination
- Beyond the Basics: Advanced Scenarios
- 1. Multiple Blocks: Interactions and Equilibrium
- 2. Plank on an Incline: Cascading Effects
- 3. Non-Uniform Plank: Varying Support and Forces
- 4. Introduction of External Forces: Pushing or Pulling
- Applications in Engineering and Physics
- Latest Posts
- Latest Posts
- Related Post
A Block Resting on a Wooden Plank: A Deep Dive into Statics and Dynamics
This seemingly simple scenario – a block resting on a wooden plank – offers a surprisingly rich landscape for exploration in physics, engineering, and even materials science. This article delves into the various aspects of this seemingly mundane situation, examining the forces at play, the factors influencing stability, and the potential for movement. We'll move beyond the basic principles to explore more complex scenarios and applications.
Static Equilibrium: The Foundation of Stability
When a block rests on a wooden plank, the primary concern is its stability. This stability is governed by the principles of static equilibrium. A body is in static equilibrium when the net force acting on it is zero, and the net moment (torque) about any point is also zero. Let's break down the forces involved:
1. Gravity: The Ever-Present Force
The most significant force acting on the block is gravity. This force, denoted by F<sub>g</sub>, acts vertically downwards, pulling the block towards the center of the Earth. The magnitude of this force is given by F<sub>g</sub> = mg, where m is the mass of the block and g is the acceleration due to gravity (approximately 9.81 m/s² on Earth).
2. Normal Force: The Plank's Resistance
The wooden plank exerts a reaction force on the block, known as the normal force (F<sub>n</sub>). This force acts perpendicular to the surface of the plank and prevents the block from falling through the plank. In an ideal scenario, where the plank is perfectly rigid and the block is perfectly rigid, the magnitude of the normal force is equal and opposite to the gravitational force: F<sub>n</sub> = F<sub>g</sub>.
3. Friction: The Unsung Hero (and Villain)
Friction plays a crucial role in determining the stability of the block. There are two types of friction to consider:
-
Static Friction (F<sub>s</sub>): This force prevents the block from moving when an external force is applied. It's a self-adjusting force, increasing up to a maximum value (F<sub>s,max</sub>) before the block starts to slide. This maximum static friction is proportional to the normal force: F<sub>s,max</sub> = μ<sub>s</sub>F<sub>n</sub>, where μ<sub>s</sub> is the coefficient of static friction between the block and the plank. The coefficient of static friction depends on the materials in contact (wood and the block material).
-
Kinetic Friction (F<sub>k</sub>): Once the block starts to move, static friction is replaced by kinetic friction. Kinetic friction opposes the motion of the block and is also proportional to the normal force: F<sub>k</sub> = μ<sub>k</sub>F<sub>n</sub>, where μ<sub>k</sub> is the coefficient of kinetic friction. Typically, μ<sub>k</sub> < μ<sub>s</sub>, meaning kinetic friction is less than maximum static friction.
Factors Influencing Stability
Several factors influence the stability of the block on the wooden plank:
1. The Coefficient of Friction: A Crucial Parameter
The coefficient of friction (both static and kinetic) is paramount. A higher coefficient of static friction implies a stronger resistance to movement, leading to greater stability. The material properties of both the block and the plank significantly impact the coefficient of friction. A rougher surface generally leads to a higher coefficient of friction.
2. The Angle of Inclination: Tipping Point
If the plank is inclined, the stability of the block is significantly affected. As the angle of inclination increases, the component of gravity parallel to the plank's surface increases, potentially overcoming the static friction and causing the block to slide. The critical angle, at which the block starts to slide, is determined by the coefficient of static friction: tan θ<sub>c</sub> = μ<sub>s</sub>, where θ<sub>c</sub> is the critical angle.
3. The Block's Shape and Size: Center of Gravity Matters
The shape and size of the block impact its stability. The center of gravity (CG) of the block plays a critical role. If the vertical line from the CG falls outside the base of support (the area of contact between the block and the plank), the block will topple. A wider, lower base of support enhances stability.
4. The Plank's Properties: Rigidity and Surface
The properties of the wooden plank itself influence stability. A flexible plank might deform under the weight of the block, potentially affecting the normal force distribution and stability. An uneven or damaged plank surface can also reduce stability.
Dynamic Considerations: Motion and Acceleration
Once the block starts moving, the scenario shifts from statics to dynamics. The forces acting on the block remain the same, but their net effect produces acceleration.
1. Newton's Second Law: F = ma
Newton's second law of motion dictates that the net force acting on the block is equal to the product of its mass and acceleration (F<sub>net</sub> = ma). If the component of gravity parallel to the plank exceeds the kinetic friction, the block will accelerate down the inclined plank.
2. Acceleration Down an Incline: Calculating Motion
The acceleration of the block down an inclined plank can be calculated using the following equation: a = g(sinθ - μ<sub>k</sub>cosθ), where θ is the angle of inclination, g is the acceleration due to gravity, and μ<sub>k</sub> is the coefficient of kinetic friction.
3. Factors Affecting Acceleration: Friction and Inclination
The acceleration of the block is directly influenced by the angle of inclination and the coefficient of kinetic friction. A steeper incline leads to higher acceleration, while a higher coefficient of kinetic friction reduces acceleration.
Beyond the Basics: Advanced Scenarios
The simple scenario of a block on a plank can be expanded upon to explore more complex situations:
1. Multiple Blocks: Interactions and Equilibrium
Imagine multiple blocks stacked on the plank. The analysis becomes more complex, involving internal forces between the blocks and the redistribution of forces. The stability of the entire system depends on the combined weight and the coefficients of friction between all surfaces in contact.
2. Plank on an Incline: Cascading Effects
If the plank itself is placed on an incline, the analysis becomes even more intricate. The forces acting on the plank must be considered, including the reaction forces from the supporting surface. The stability of the block and the plank becomes interdependent.
3. Non-Uniform Plank: Varying Support and Forces
A non-uniform plank with varying thickness or density introduces variations in the normal force distribution. The stability of the block is affected by the location of its center of gravity relative to the changing support provided by the plank.
4. Introduction of External Forces: Pushing or Pulling
The introduction of external forces, such as pushing or pulling the block, adds another layer of complexity. The direction and magnitude of the external force affect the net force acting on the block and can cause it to slide or topple, depending on the relationship between the external force and the friction force.
Applications in Engineering and Physics
The principles explored here have far-reaching applications in various fields:
-
Structural Engineering: Understanding static and dynamic equilibrium is crucial for designing stable structures. The analysis of forces and stability, as exemplified by the block and plank scenario, is fundamental in structural design and analysis.
-
Mechanical Engineering: The concepts of friction, force, and motion are essential for designing and analyzing mechanical systems. Understanding friction is vital in the design of machine components and the efficiency of mechanical processes.
-
Robotics: Robots often interact with objects, and understanding the forces involved, including friction and gravity, is critical for precise manipulation and control. The principles of static and dynamic equilibrium are essential in robotics to ensure stability and controlled movement.
-
Physics Education: The simple scenario of a block resting on a wooden plank serves as an excellent introductory example for illustrating fundamental concepts in statics and dynamics.
This article provides a comprehensive overview of the physics involved in a seemingly simple scenario. The principles discussed here lay the foundation for understanding more complex systems and are applicable across various engineering and scientific disciplines. Further exploration into specific scenarios, material properties, and advanced mathematical models can unlock a deeper understanding of the intricate interactions between forces and equilibrium.
Latest Posts
Latest Posts
-
Draw A Mechanism For The Following Reaction
Apr 01, 2025
-
State Farm Staff Agreement Assessment Test Answers Pdf
Apr 01, 2025
-
Introduction To Public Health 6th Edition Pdf
Apr 01, 2025
-
You Must Encrypt Files With Any Of These Extensions
Apr 01, 2025
-
Which Of The Following Pairs Of Terms Is Mismatched
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about A Block Is Resting On A Wooden Plank . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
